Area and perimeter

There are only a few formulas that you will need to memorize in order to confidently solve area problems:

Area of parallelogram (square, rectangle)

$\text{length}\ast \text{width}$ or $\text{base}\ast \text{height}$

Area of triangle

$\frac{1}{2}\ast \text{base}\ast \text{height}$

Area of circle

$\pi \ast {\text{radius}}^2$

It’s best to think of a clever way to remember these formulas. For instance, you can see that a triangle is just half of a rectangle (or a parallelogram):

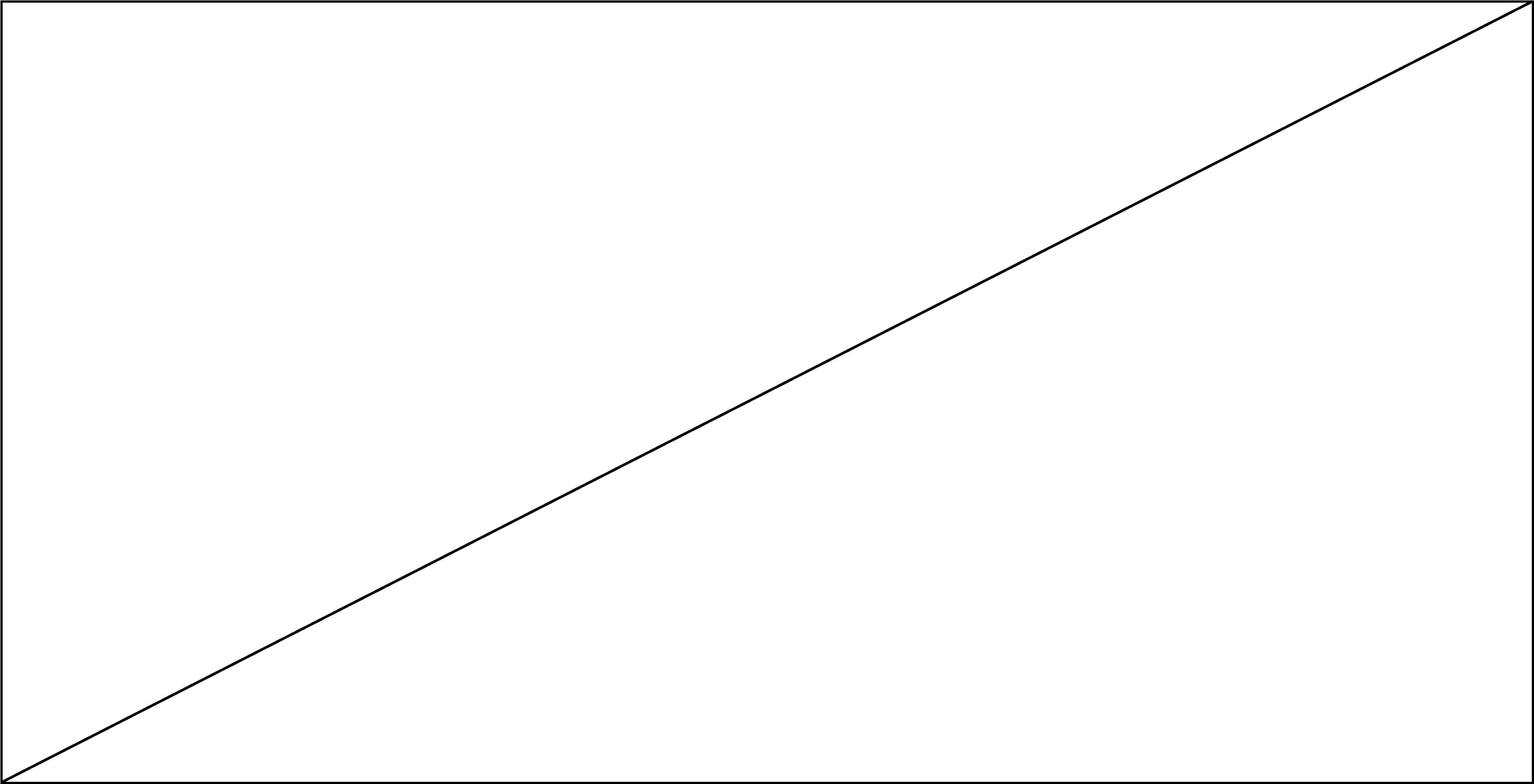
So this can be a good reminder that the area of a triangle is one-half $\times$ base $\times$ height!

Once you are comfortable with these formulas, then we can dive further into the kinds of properties you may see on the test.

Properties of shapes

Let’s first examine the different kinds of triangles: equilateral, isosceles, and scalene triangles. Remember, all triangles follow the area formula above for triangles (base $\times$ height $\times \frac{1}{2}$).

Definitions

Equilateral triangles

Triangles where all three sides are equal to each other.

Isosceles triangles

Triangles where two sides are equal to each other and one is not.

Scalene triangles

Triangles where no sides are equal to each other.

On to parallelograms! This may seem like easy stuff, but it’s important to recognize the terms for when they eventually come up on a test. Remember, all parallelograms follow the area formula above for parallelograms (length $\times$ width or base $\times$ height).

Definitions

Parallelogram

A four-sided shape where opposing sides are equal. Rectangles, squares, and rhombi are all parallelograms.

Rectangle

A four-sided shape where opposing sides are equal and all four angles are right angles.

Square

A four-sided shape where all sides are equal and all four angles are right angles. A square is a rectangle, but a rectangle is not a square because a rectangle does not require that all sides are equal while a square does.

Unfamiliar shapes

You may be presented with a strange-looking shape and be asked to find its area. These questions seem very difficult, as if there is some formula that you have forgotten. However, this strategy will save you every time as long as you remember the formulas for parallelograms, triangles, and circles.

When you must find the area of an unfamiliar shape, try to draw lines (or remove lines) in order to form shapes that you are familiar with. Trapezoids are the most common example that appear on the test.

How do you find the area of a trapezoid? Do you remember the formula? Probably not. So, follow this strategy to break it up into familiar shapes:

We draw two lines, breaking up the trapezoid into a rectangle and two equal triangles. If we remember our basic formulas above, then finding the area of these shapes should be simple! So, this is the strategy: break down unfamiliar shapes into basic, familiar shapes by drawing or removing lines.

Partial areas

There are many problems that will ask you to find the area of part of a shape, often in the form of a word problem. Usually, this will be in the form of putting a shape within a shape, like below:

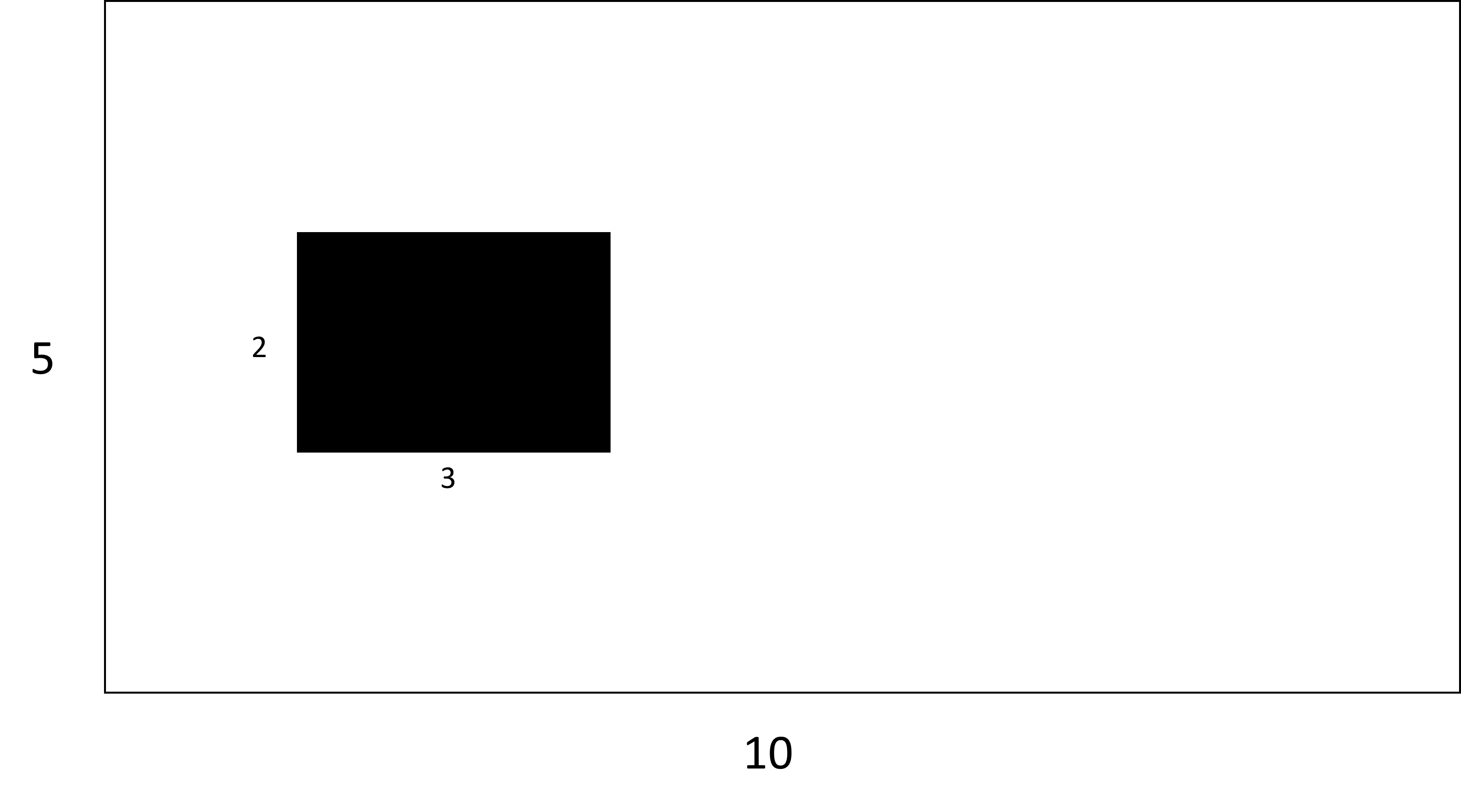
The question associated with this shape could be that this is a wall with a window that is represented by the shaded rectangle. What is the area of the surface of the wall? In this case, we do not want the area of the window included in the area of the wall. So, we find the total area of the shape, then subtract the portion we don’t want.

For this problem specifically, we would find the area of the whole rectangle (length $\times$ width)

$$5\ast 10=50$$

Then, we find the area of the window, the portion we don’t want to be included in the answer (length x width).

$$2\ast 3=6$$

Finally, subtract the part we don’t want from the total. We will end up with just the portion we want.

$$50-6=44$$

Area using algebra

Basic algebra questions involving area can be solved by simply knowing the formulas listed in this chapter. For instance:

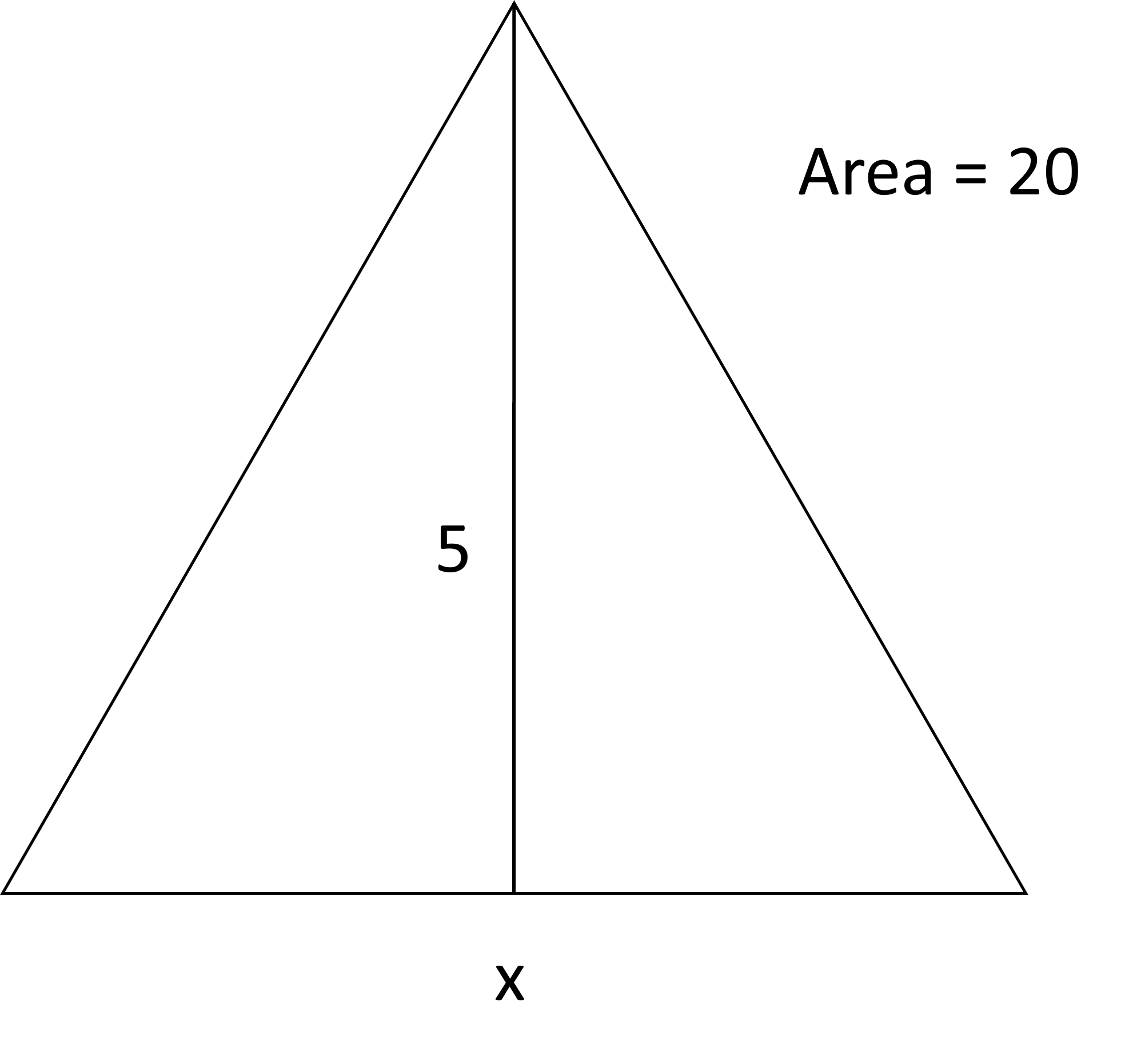
Try solving this one on your own, knowing the triangle formula.

Word problems involving area are often more difficult for most students than these basic algebra questions. You are expected to write out the formula as we did above, but using $x$, as you will see.

Suppose this is the word problem:

The length of a rectangle is twice as large as its width. If the area is $8$, what is the length of the rectangle?

Let’s start with the formula we know, Area $=$ length $\times$ width.

Now, let’s plug in the information we have been given. Area $=8$, length $=2\times$ width (twice width), and width $=$ whatever the width is ($W$). So let’s write it all together:

$$8=2W\ast W$$

Now we can continue with our algebraic operations to find the one variable in the equation:

$$\begin{array}{rl}8/2& =W\ast W\\ 4& =W^2\\ \sqrt{4}& =2\\ 2& =W\end{array}$$

Lastly, we find what the question wanted: length. Length = 2W, so length = 2(2) = 4.

Perimeter

Perimeter is the total length of the outside of a shape. The only exception is the perimeter of a circle, which is actually called the circumference. For the most part, you will not need to memorize any formulas for the perimeter. However, there are a few to have in mind:

Circles

The perimeter (circumference) of a circle is $2\pi \times \text{radius}$ (not to be confused with the area formula, $\pi \times {\text{radius}}^2$).

Squares

Since each side is equal, the perimeter would be $4$ times one side, or $4L$.

Rectangles

Rectangles have $2$ lengths and $2$ widths that are equal to each other, making the perimeter $(2\times L)+(2\times W)$.

Triangles

Remember that in order to find the hypotenuse of a right triangle, you need to use the Pythagorean Theorem $(a^2+b^2=c^2)$. This will be handy when solving for the perimeter of a right triangle.

Don’t panic. Remembering the equations for perimeter is largely intuitive. If you had to you most like could think them out logically without having to memorize them (except for circumference, $2\pi r$. Memorize that one!). So, the perimeter should largely be a matter of problem-solving, while the area is more a matter of memorization.

Geometry questions involving area and perimeter will often use both in the same question, requiring you to do some algebra. Let’s look at an example of when they may be combined in the form of an algebraic question:

The area of a rectangle is $8$ and its perimeter is $12$. What are the values of the lengths of the rectangle? Try solving this out, then review your answer. Start by using the area and perimeter formulas for both area and perimeter and trying to relate them to each other.

a. 8 & 4
b. 2 & 4
c. 8 & 1
d. 3 & 4
e. 6 & 2

Be careful! This method is the easy way out. Not all questions will be this easy to solve, so it is worth reviewing the proper way to solve this problem, by a system of equations and quadratic equations. Please recap the [Solving a system of equations] and [Factorization of quadratics and cubics] chapters if you are not confident with them!

Here is the proper way to solve this problem by a system of equations:

List out the two equations we will be using, and plug in the numbers we are given:

$$\begin{array}{rl}\text{Area}& =\text{base}\ast \text{height}\\ 8& =b\ast h\end{array}$$

$$\begin{array}{rl}\text{Perimeter}& =2\ast \text{base}+2\ast \text{height}\\ 12& =2\ast \text{base}+2\ast \text{height}\\ 12& =2b+2h\end{array}$$

Now we solve one equation for one variable in order to get rid of them!

Using the area equation:

$$\begin{gathered} 8=b\ast h \\ b=\frac{8}{h} \end{gathered}$$

Plug in the new value for the base into the final equation.

Using the perimeter equation:

$$12=2(\frac{8}{h})+2h$$

Multiply into the parentheses:

$$12=\frac{16}{h}+2h$$

Solve for $h$ by getting the first $h$ out of the fraction:

$$12-2h=\frac{16}{h}$$

Multiply by $h$:

$$h(12-2h)=16$$

Distribute through the parentheses:

$$12h-2h^2=16$$

Rewrite as a quadratic equation:

$$-2h^2+12h-16=0$$

Solve for $h$ by quadratic formula or by factoring. We will factor, but first, reduce the equation by dividing by $-2$ to make it easier to work with. We want the first term to look clean:

$$h^2-6h+8=0$$

Factor:

$$(h-4)(h-2)$$

Answers:

$$h=4\text{ or }h=2$$

Since we are looking for the base AND the height, and the height could be either of these values, these two values are the answer!

Key points

Area formulas. Memorize the formulas above for circles, parallelograms, and triangles.

Shape properties. Properties of scalene (no similar sides), isosceles (two similar sides), and equilateral triangles (all similar sides) as well as parallelograms (opposing sides equal), rectangles (opposing sides equal, four right angles), and squares (all sides equal, four right angles).

Unfamiliar Shapes. Draw or remove lines to see unfamiliar shapes as more familiar ones, like triangles and rectangles in a trapezoid.

Partial Areas. If you are only looking for part of a whole area, subtract the area of the unwanted portion from the total area.

Area Using Algebra. Plug the information you are given from a word problem into the formulas you know. You may need to replace length and width with a short equation using $x$, then solve for that variable.

Perimeter. Keeping in mind that most of the equations can be thought up without memorizing them, but you need to know the formulas for circumference ($2\pi r$) and the Pythagorean Theorem ($a^2+b^2=c^2$).