Rhombus geometry | Plane geometry | ACT Math

A rhombus is a parallelogram where all sides are equal. It is essentially just a square that has been slightly skewed. Some geometric properties for a rhombus are the same as for a square, but others are not. We will review these properties in this section.

Geometric properties

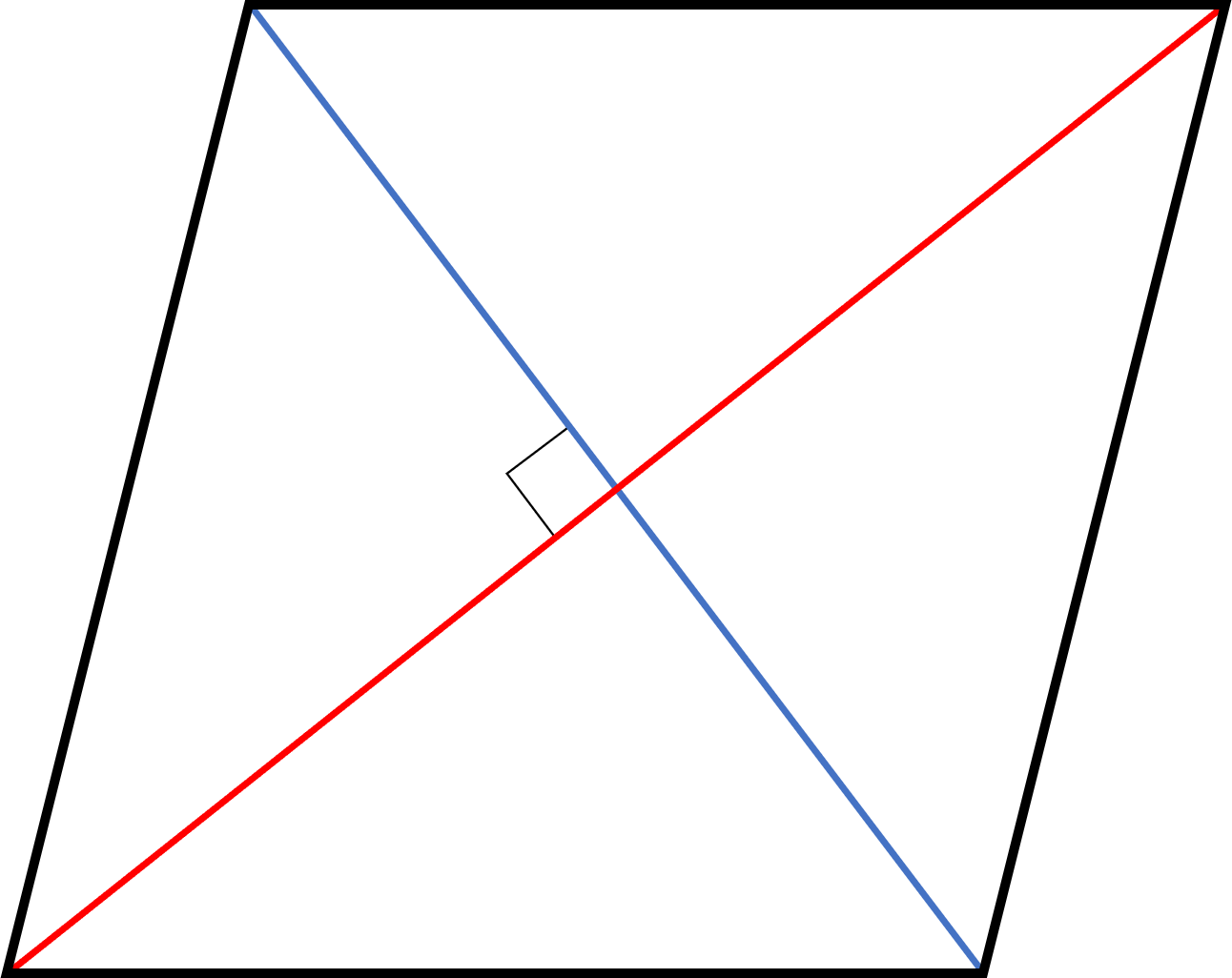
Since a rhombus is a parallelogram, we know that opposing sides are parallel. This means that the bottom and top lines are parallel and the left and right lines are parallel.

All four sides of a rhombus are equal in length, but the shape is slightly askew. So, just like how we say that opposing sides are parallel, we may also say that opposing angles are congruent.

Just like a square, the sum of all angles inside a rhombus must add up to 360 degrees. Since a rhombus has equal opposing angles, we know that any two adjacent angles add up to 180 degrees.

Lastly, the two diagonal lines drawn from corner to corner within a rhombus intersect perpendicularly

The following images of a rhombus show each of these properties:

$Rhombus with opposite equal congruent angles$

$Rhombus with opposite parallel sides$

Area of a rhombus

The area of a rhombus may be calculated using two primary methods. Either method works just fine, so it is up to you to determine which method you should use according to the information you are given.

Parallelogram method

This method takes into account that a rhombus is a parallelogram, and thus its area can be calculated as such. The area of a parallelogram is below:

$A = base * height$

So, if we know the height (sometimes called the altitude) of the rhombus, we can use the same formula. It is important to note that you do not multiply the base by the side. The side is slanted, causing it to be a bit longer than the height of the rhombus. Thus, the area of a rhombus is given by the following formula:

$A = base * height$

Diagonal method

This method uses the length of the diagonal lines to find the area of the rhombus. The two diagonals are not equal in length. If you are given the length of the diagonal lines, the formula for the area of a rhombus is as follows:

$A = (\frac{1}{2}) * diagonal_{1} * diagonal_{2}$

Examples

Try out the following examples by first determining the best method to use and then finding the area of the rhombus.

Given the following rhombus with diagonal lengths of $5$ and $6$ , what is the area?

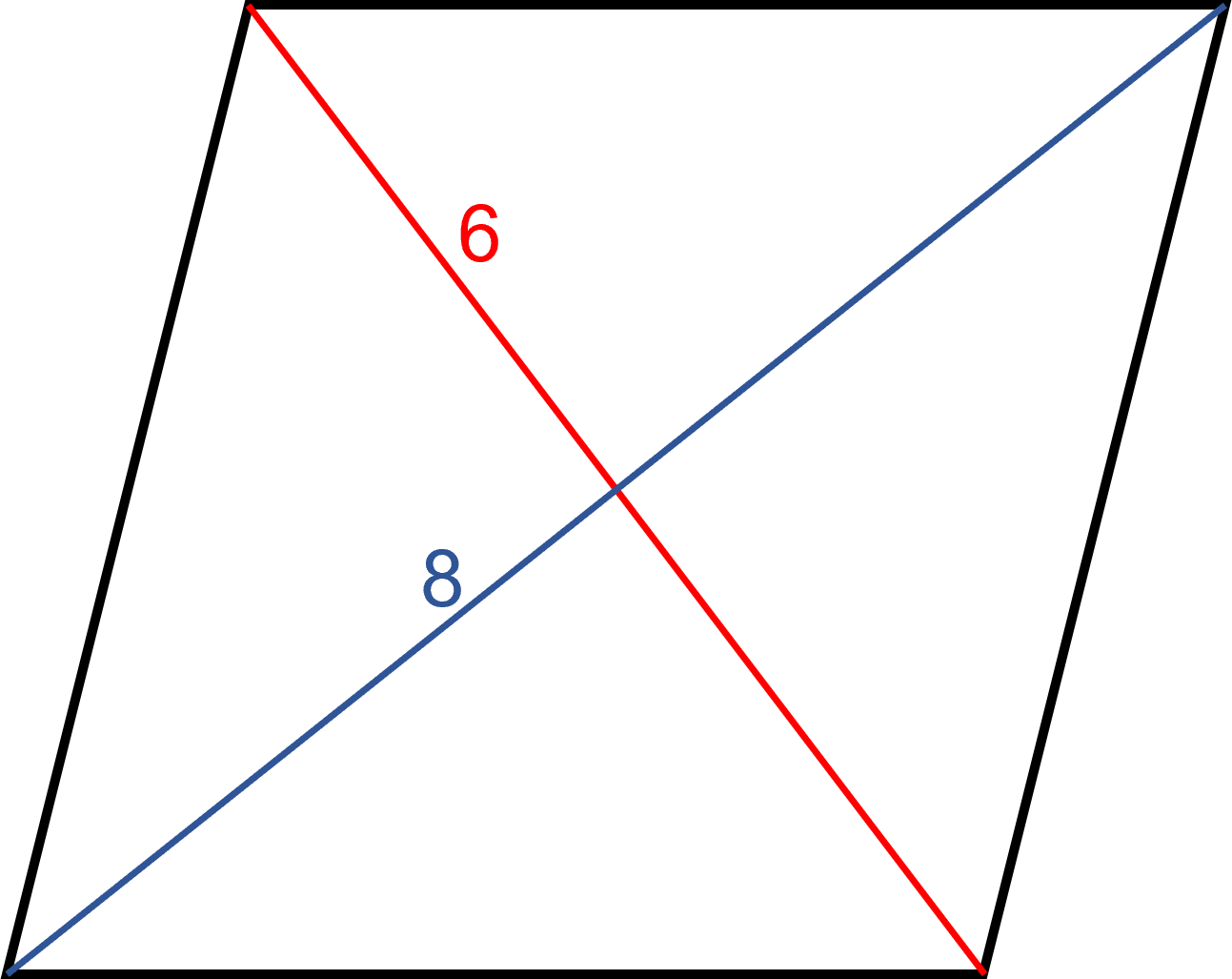
$Calculate area of a rhombus given length of diagonals$

(spoiler)

Use the diagonal method:

$A A A = (\frac{1}{2}) * diagonal_{1} * diagonal_{2} = (\frac{1}{2}) * 6 * 8 = 24$

The area of the rhombus is $24$ .

What is the area of the following rhombus that has a side length of $5$ and a height of $4$ ?

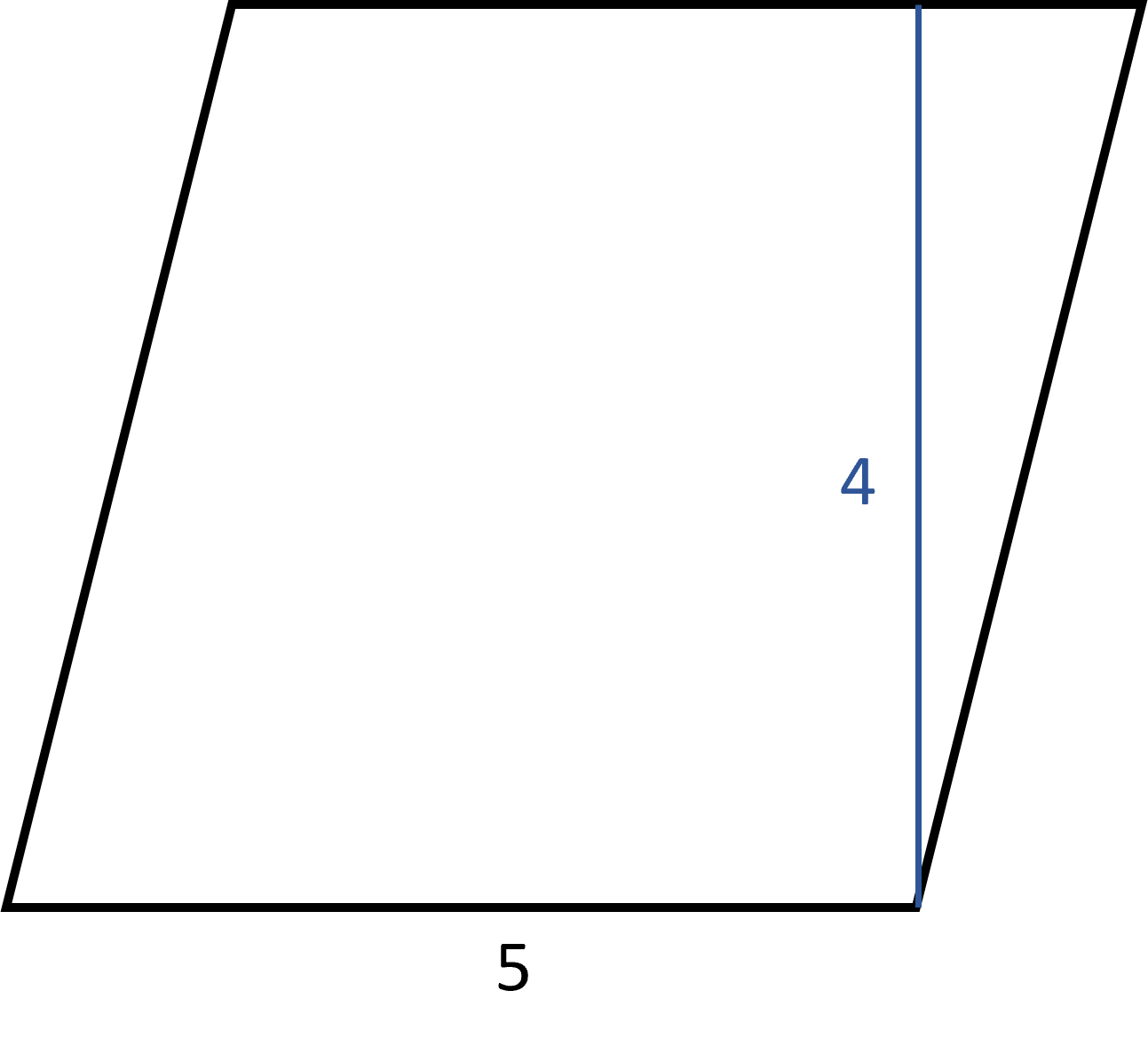
$Calculate area of a rhombus given length of sides$

(spoiler)

Use the parallelogram method:

$A A A = base * height = 5 * 4 = 20$

The area of the rhombus is $20$ .

Key points

Rhombus. A rhombus is a square that sits askew.

Parallel sides. Opposing sides of a rhombus are parallel to each other.

Equal angles. Opposing angles in a rhombus are congruent.

Sum of angles. The angles in a rhombus sum up to 360 degrees, and any two adjacent angles sum up to 180 degrees.

Diagonals. The diagonals of a rhombus intersect perpendicularly.

Area (parallelogram method). $A = base * height$ (NOT $base * side$ )

Area (diagonal method). $A = (\frac{1}{2}) * diagonal_{1} * diagonal_{2}$