You have likely seen many problems that include equations that look something like this:
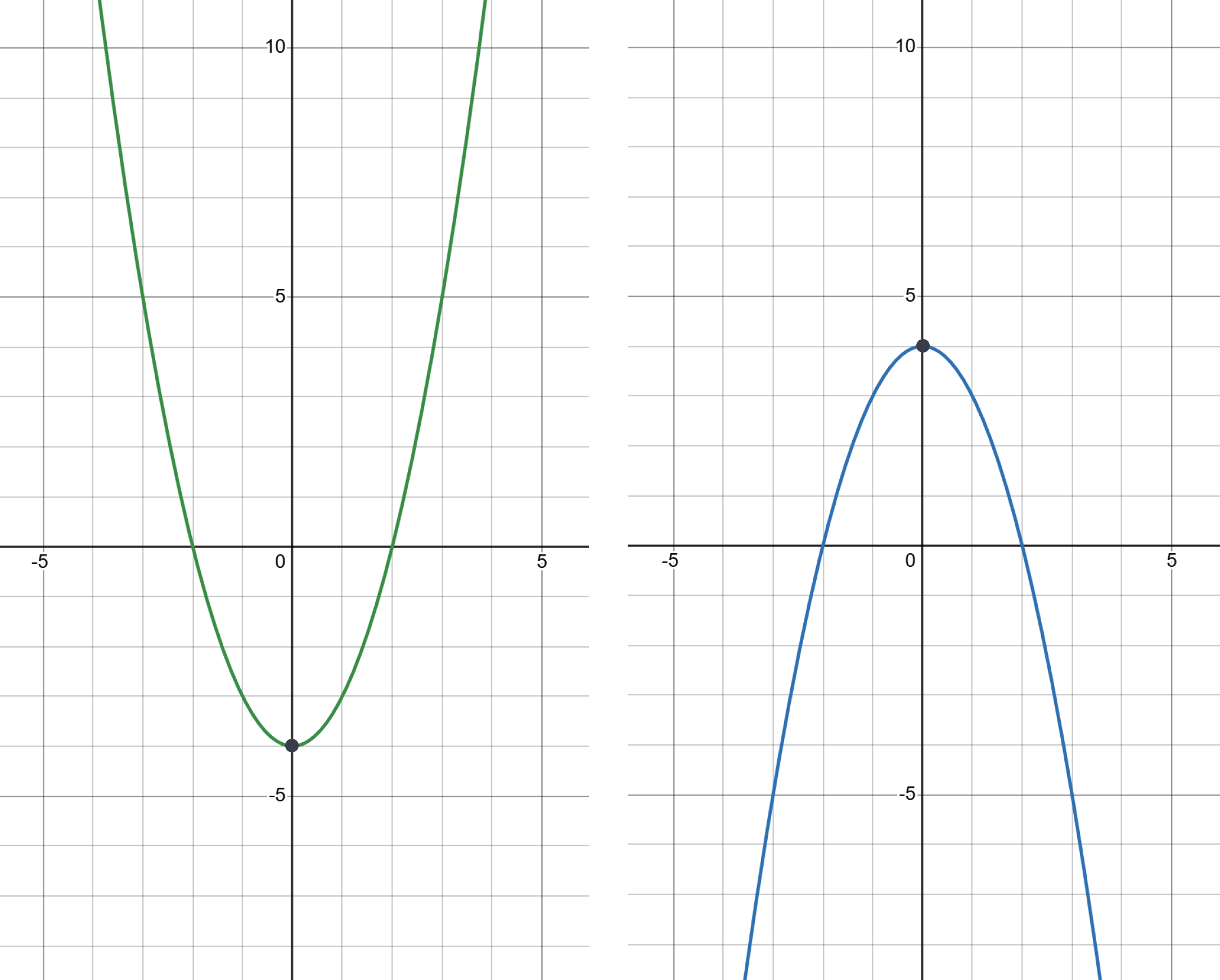
You also probably have experience solving for the roots of an equation, which is just the value of when equals . When you imagine the equation above on an - coordinate plane, the equation is represented by a parabola, and the parabola’s -intercepts are the roots of the equation. Parabolas can look something like either of these two graphs, where the first represents a quadratic equation when the variable ‘’ is positive and the second, downward parabola, reflects a quadratic equation with a negative ‘’ value. The vertex of either parabola is shown by the point.
Just like an equation for a line, you can plug in any value for and solve for the coordinate along that line, and vice versa. And just like the equation for a straight line, the value at the end of the equation represents the -intercept.
is the -intercept
is the -intercept
If seeing curved lines brings back nightmares from calculus, don’t worry! You are not required to use calculus in the GRE. Most questions that involve a parabola, either drawn out or just in its equation form, will involve either finding an intercept, finding a specific point along the parabola, finding where lines intersect, or shifting a parabola on the plane. All of these can be solved by treating the parabola similarly to how you would treat a straight line; however, let’s take a little bit of time to review.
A point of a parabola can be found by replacing either the or value with a number, and then solving for the other variable. The value you find for the other variable, combined with the original value you chose, make up the ordered pair. If you found two solutions, it just means that there are two points that have the same coordinate.
What is the value of ‘’ in the ordered pair that exists on the parabola
Answer:
To find the -intercept, simply set equal to zero and solve for . To find the -intercept, set equal to zero and solve for .
What is the -intercept of
Answer: the -intercept is
To find where a parabola and a line intersect, set them both equal to y and then set them equal to each other. This allows you to solve for the coordinate where they intersect. Once you have the coordinate, plug it into either equation to solve for the coordinate.
At what two points do and intersect?
Answer:
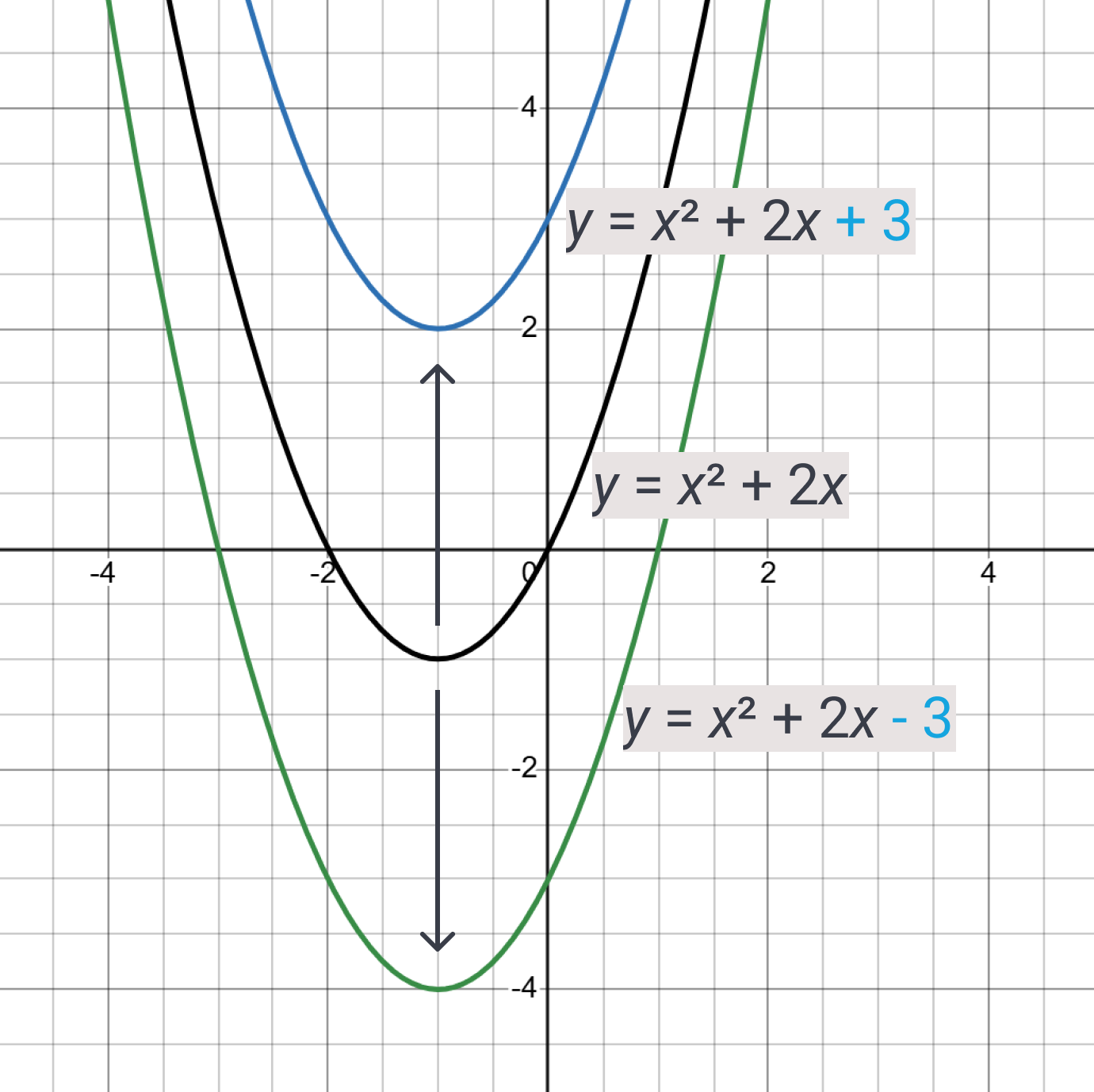
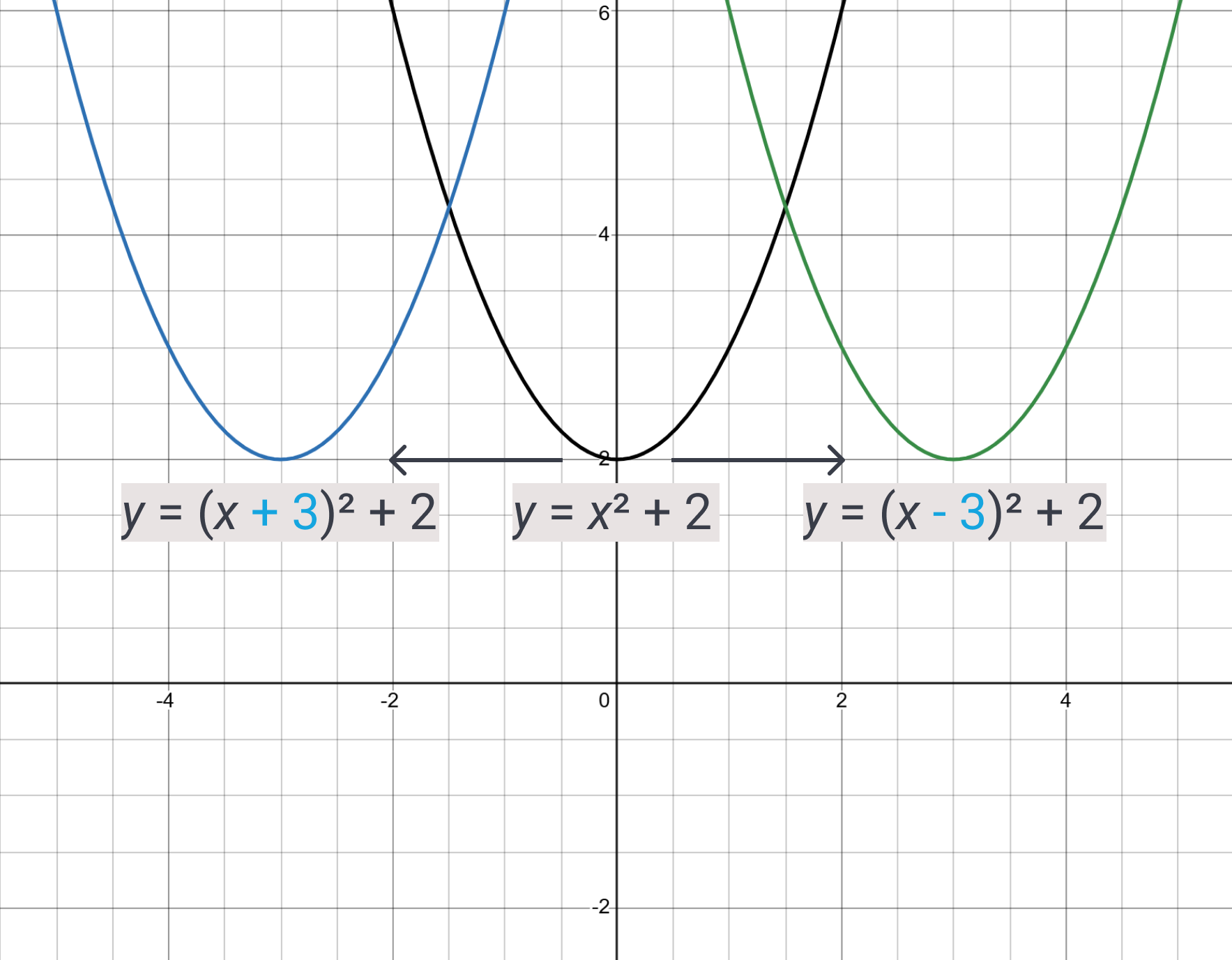
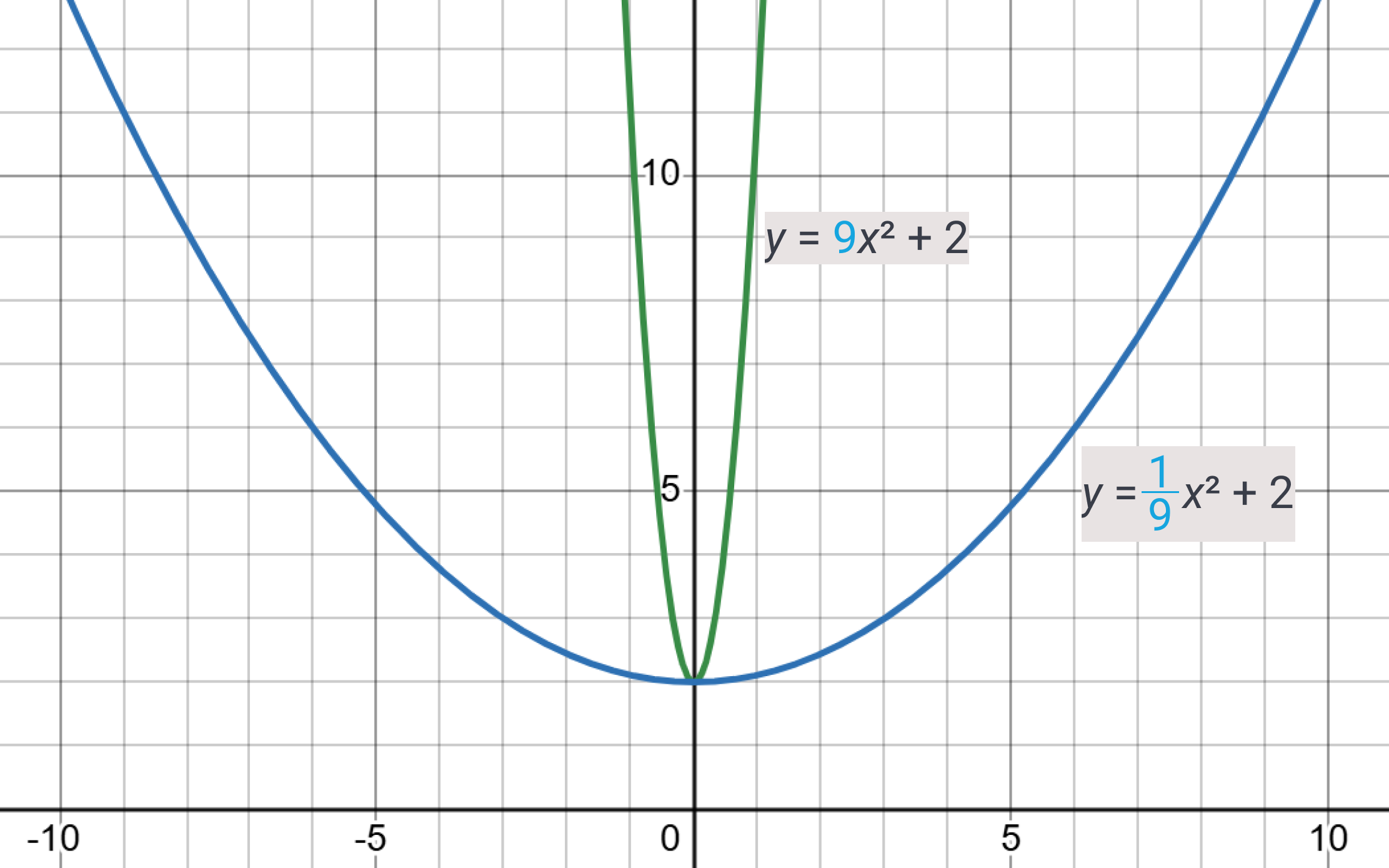
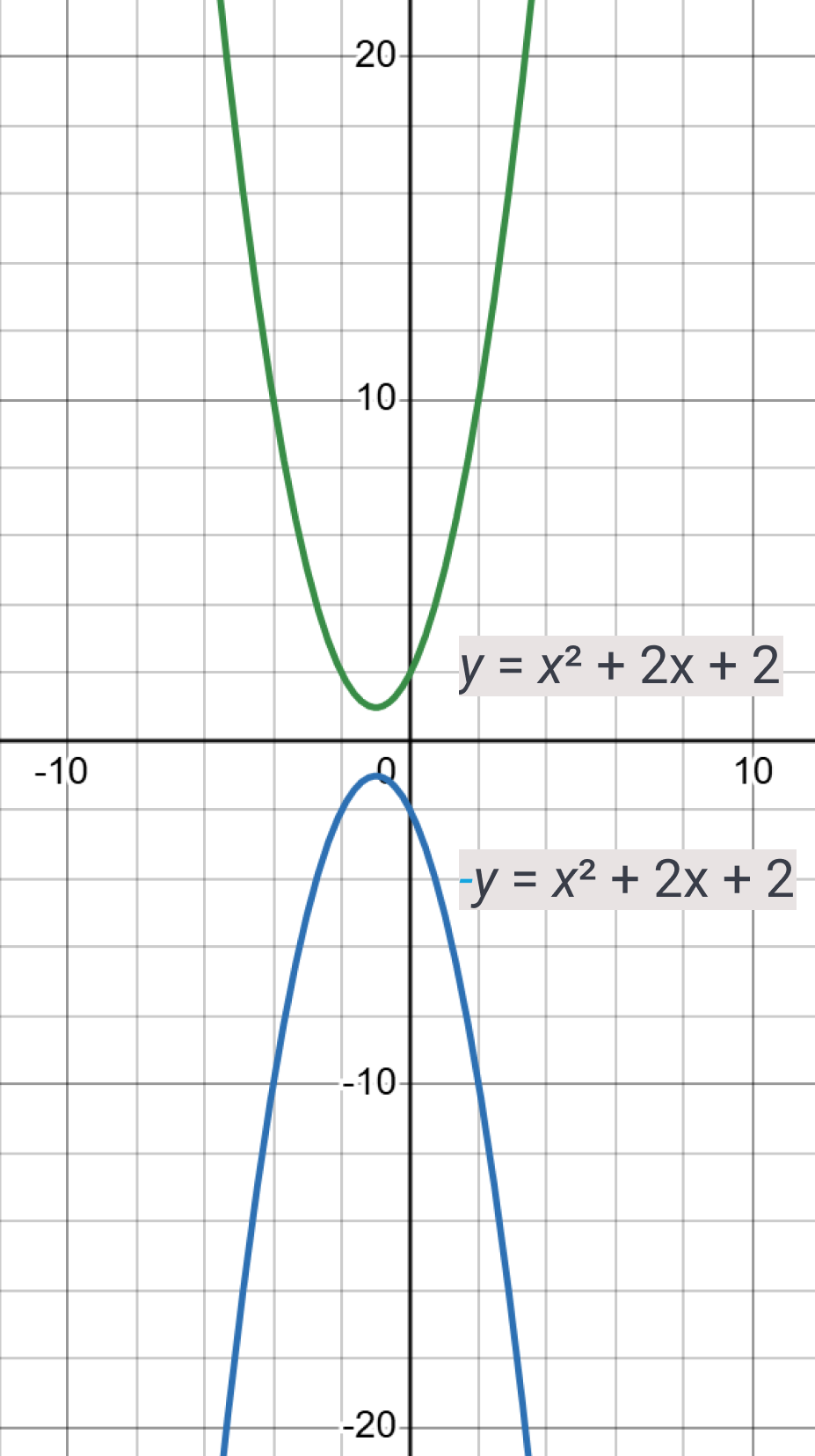
Changing any part of the equation of a parabola can change the shape and location of the parabola in different ways. Here is a table showing all of the ways a parabola can be changed, and what needs to be done to the equation to make such a change. This is not commonly tested by the GRE but it still may come up in the exam.
