Graphing inequalities | Geometry | Quantitative reasoning

As you already know, an equation that involves $x$ and $y$ variables can be plotted as a line on a coordinate plane. What if we switch out the equal sign for an inequality? The line on the graph would not change, but the meaning of the graph would. With inequalities, the possible values of $x$ and $y$ do not lie on a single line, instead, they lie in a region either above or below the line. Here is an inequality, written similarly to the $y = m x + b$ format. The only difference is that the possible pairs of $x$ and $y$ all occur above the line, instead of on it.

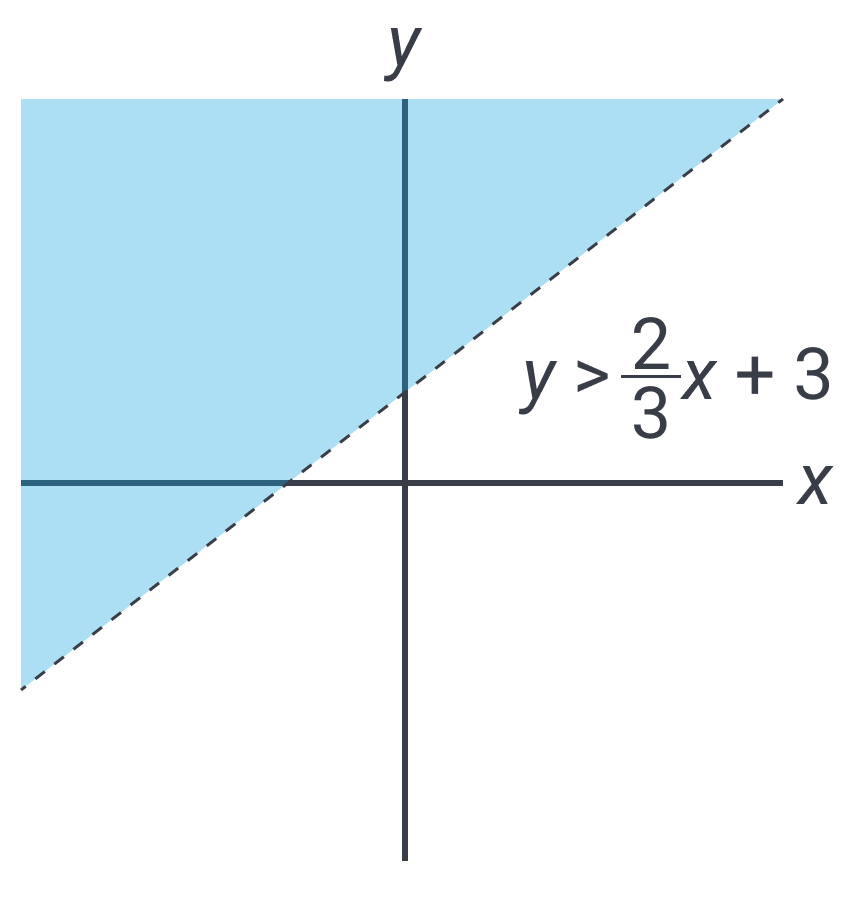
graphing inequalities 01

It is actually pretty easy to determine which side of the partition should be shaded. Begin by making sure the inequality is written similarly to the $y = m x + b$ format. If the inequality is $>$ or $\geq$ then the shading should be on the top half of the line. If the inequality is $<$ or $\leq$ then the shading should be the bottom half.

Sidenote

Dotted line vs. solid line

This is relatively unimportant for the GRE, but a dotted line represents $>$ or $<$ while a solid line represents $\geq$ or $\leq$ . When the inequality includes “or equal to” then the coordinate pairs could also exist on the line.

Try to match each inequality with a graph drawn below.

$y > 2$

$y > x$

$y \leq - 2 x - 2$

$y \geq - 2 x - 2$

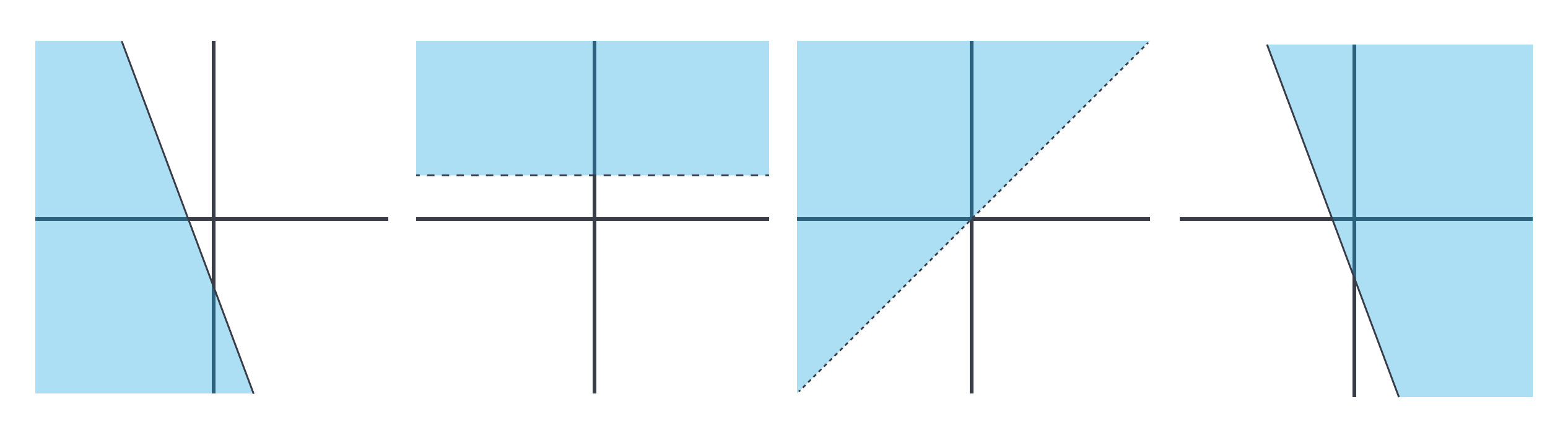
graphing inequalities 02

(spoiler)

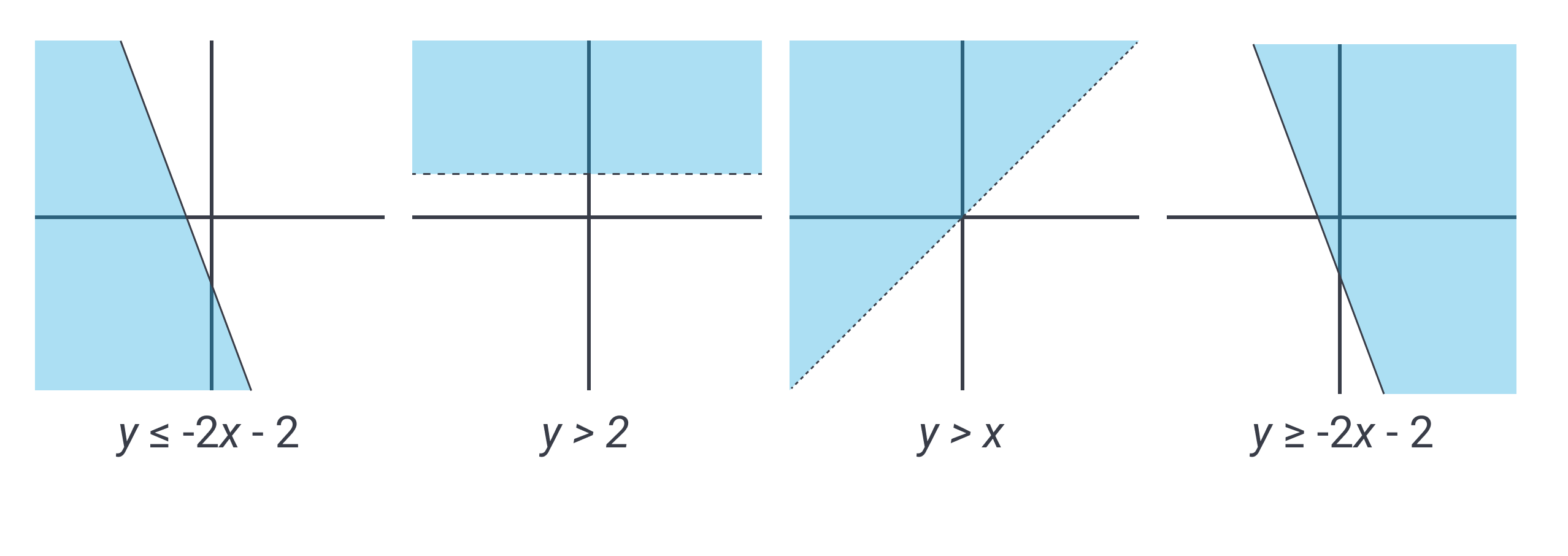
Answers:

graphing inequalities 03

Now try this GRE-level practice problem that utilizes the concept of graphing inequalities.

In which quadrants does the intersection of both inequalities exist? (Select all that apply.)

$y \leq 3 x + 2$
$y \leq x - 4$

A. Quadrant I
B. Quadrant II
C. Quadrant III
D. Quadrant IV

(spoiler)

Answer: A, C, D

Explanation:

Start by graphing both inequalities.

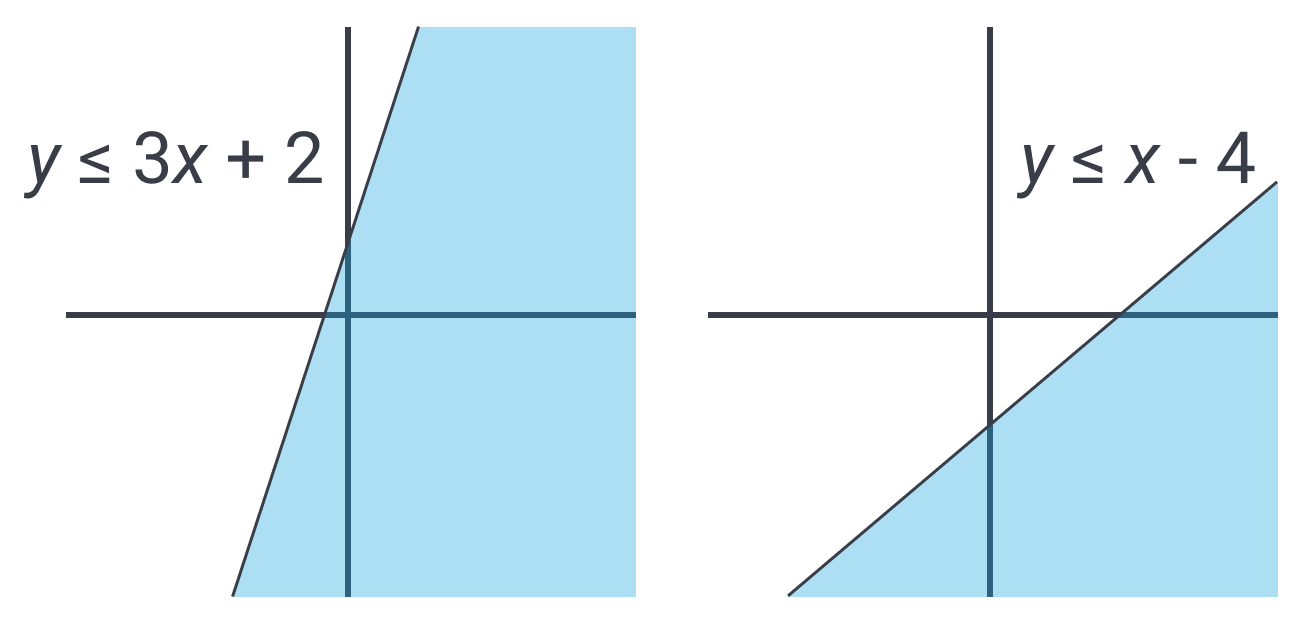
graphing inequalities 04

The first graph’s shaded region is in all four quadrants (if you look closely, you can see that it barely touches quadrant II), while the second graph’s shaded region is only in quadrants I, III, and IV. The intersection would be the quadrants that both graphs share. Thus, the answer should be quadrants I, III, and IV.

For context, the union of the quadrants represents all possible quadrants that either inequality could be in. If the question asked for the union instead of the intersect, all four answer choices would be correct.