Nonlinear functions and graphs | Coordinate geometry | ACT Math

There are many nonlinear functions for which you will need to be familiar with their graphs. The graphs below will show the parent functions for these common nonlinear functions. The parent function is the simplest form of a certain function.

We will review how these parent functions normally look graphically and mathematically, be able to describe limitations in the form of asymptotes, and learn how to predict what a graph will look like after altering the parent function.

Quadratic

$y = x^{2}$

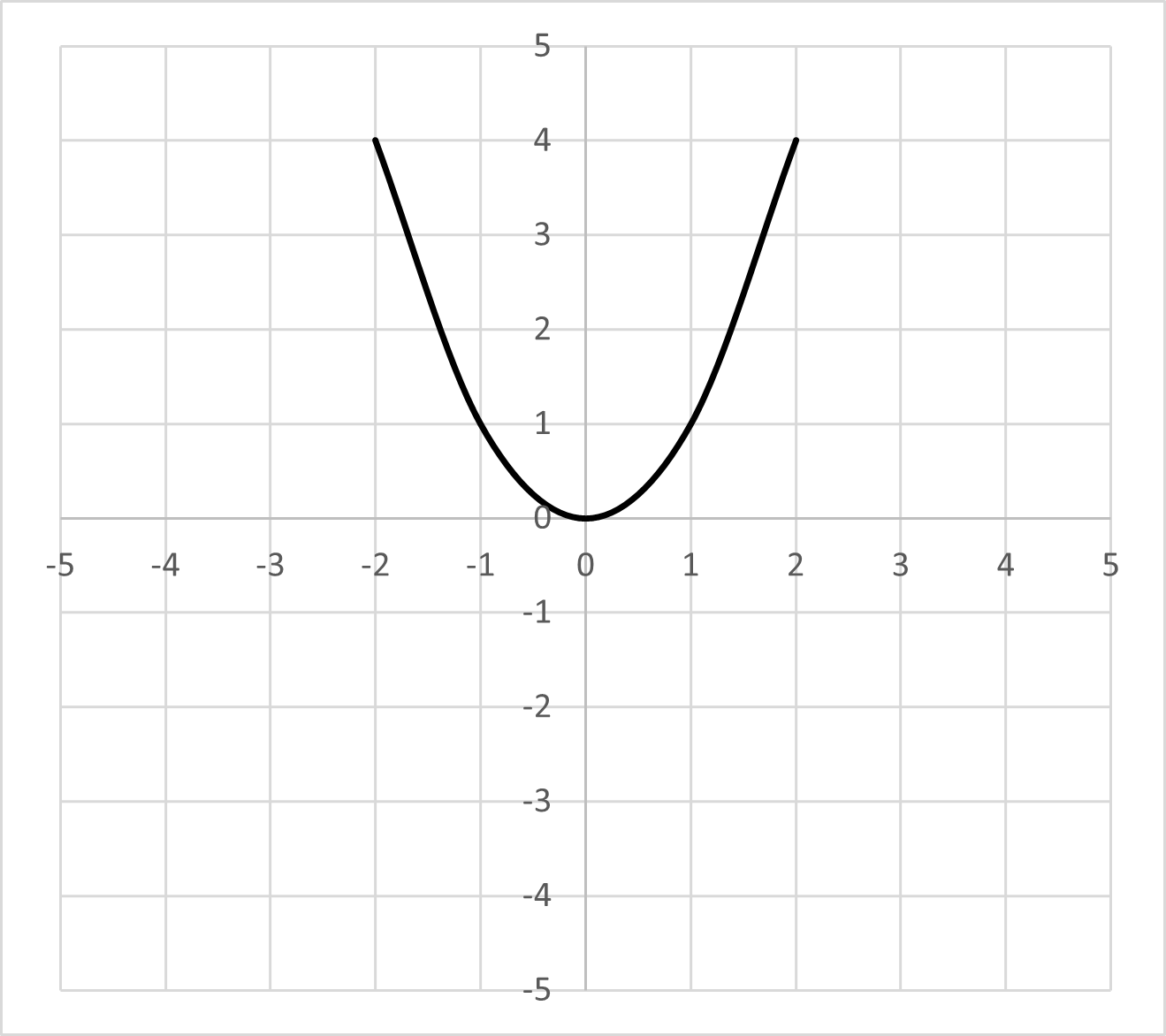
$Graph of nonlinear function parabolic quadratic equation$

Absolute value

$y = ∣ x ∣$

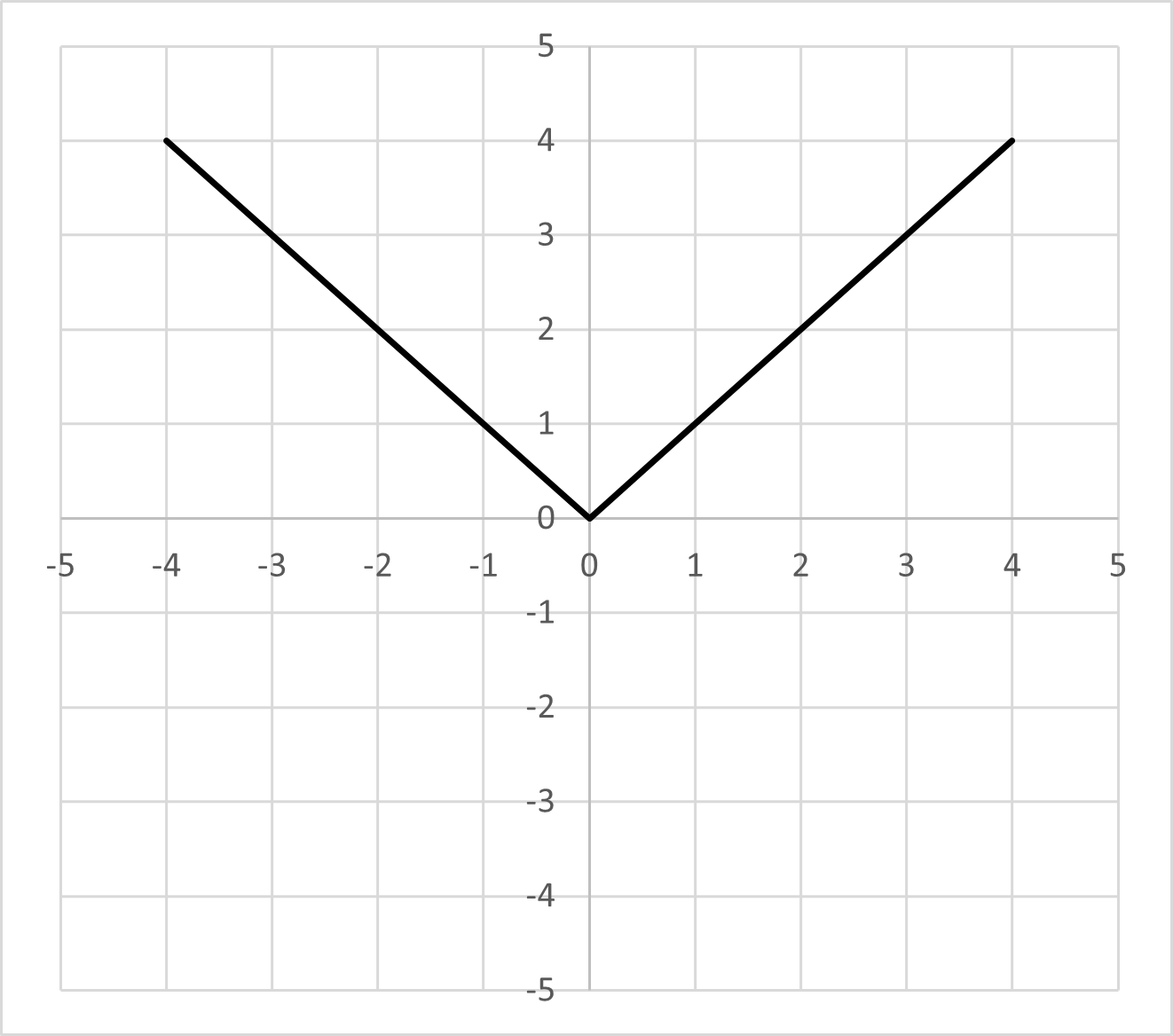
$Graph of nonlinear function absolute value equation$

Cubic

$y = x^{3}$

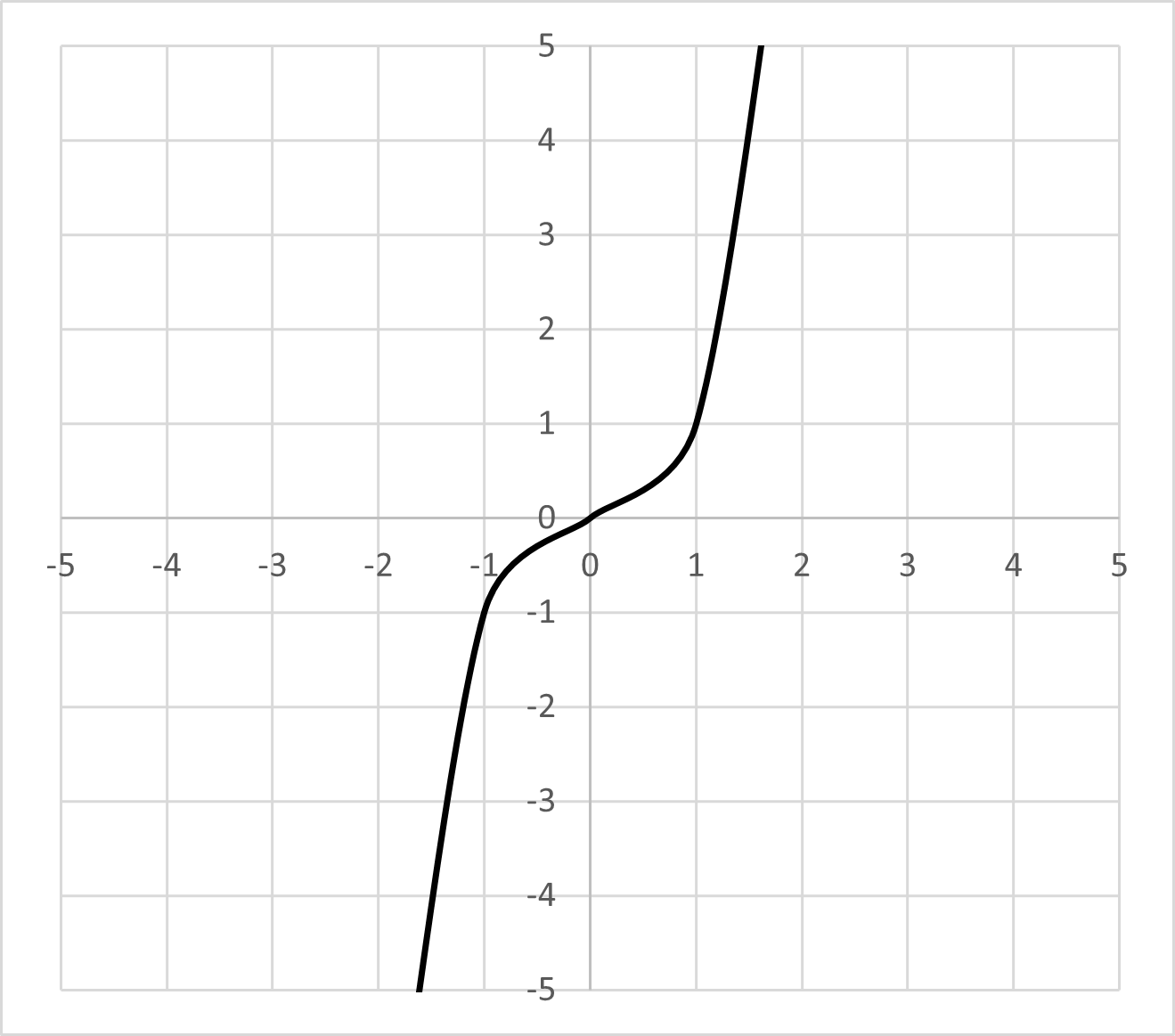
$Graph of nonlinear function cubic equation$

Exponential

$y = e^{x}$

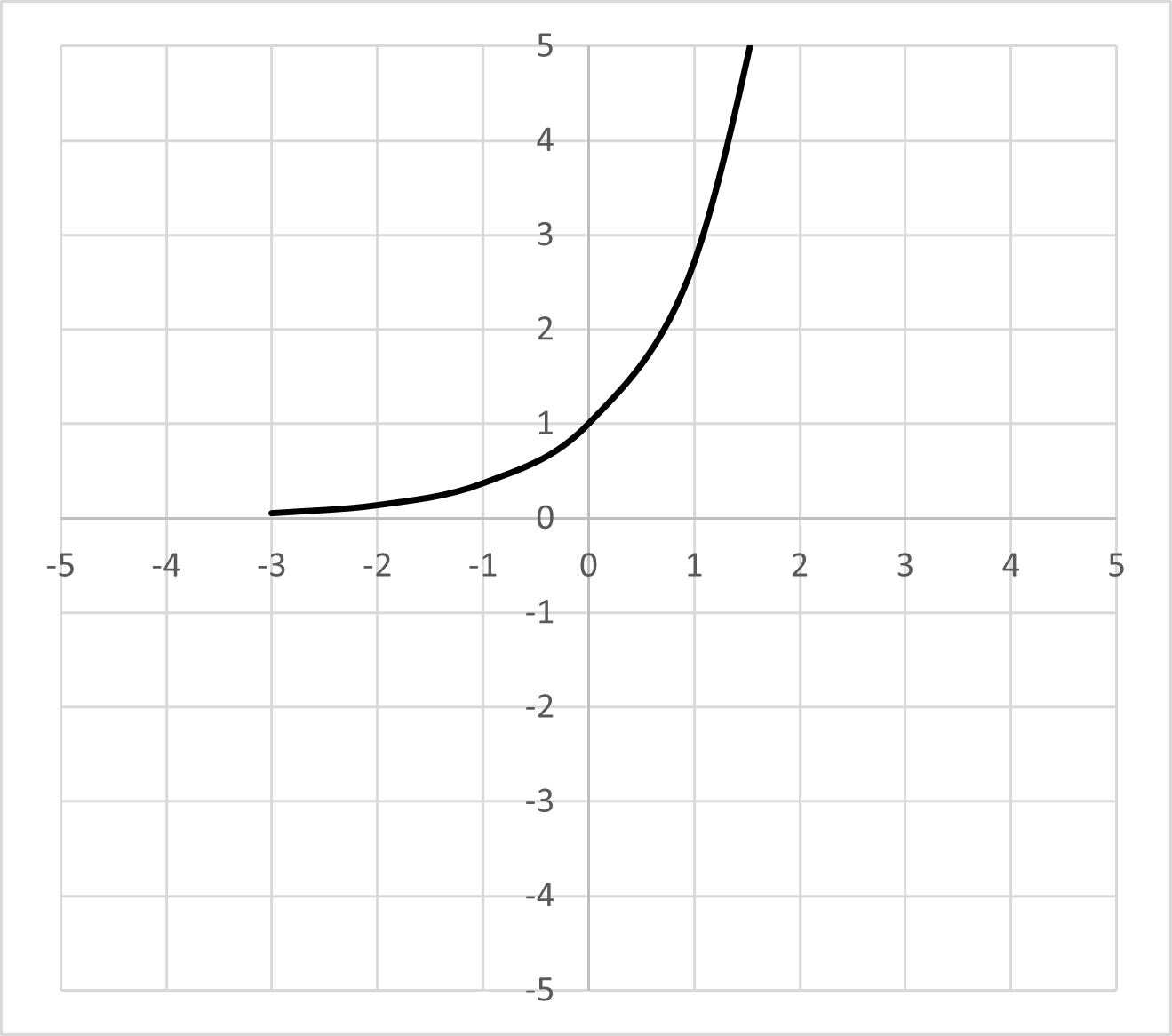
$Graph of nonlinear function exponential equation$

Logarithmic

$y = l o g (x)$

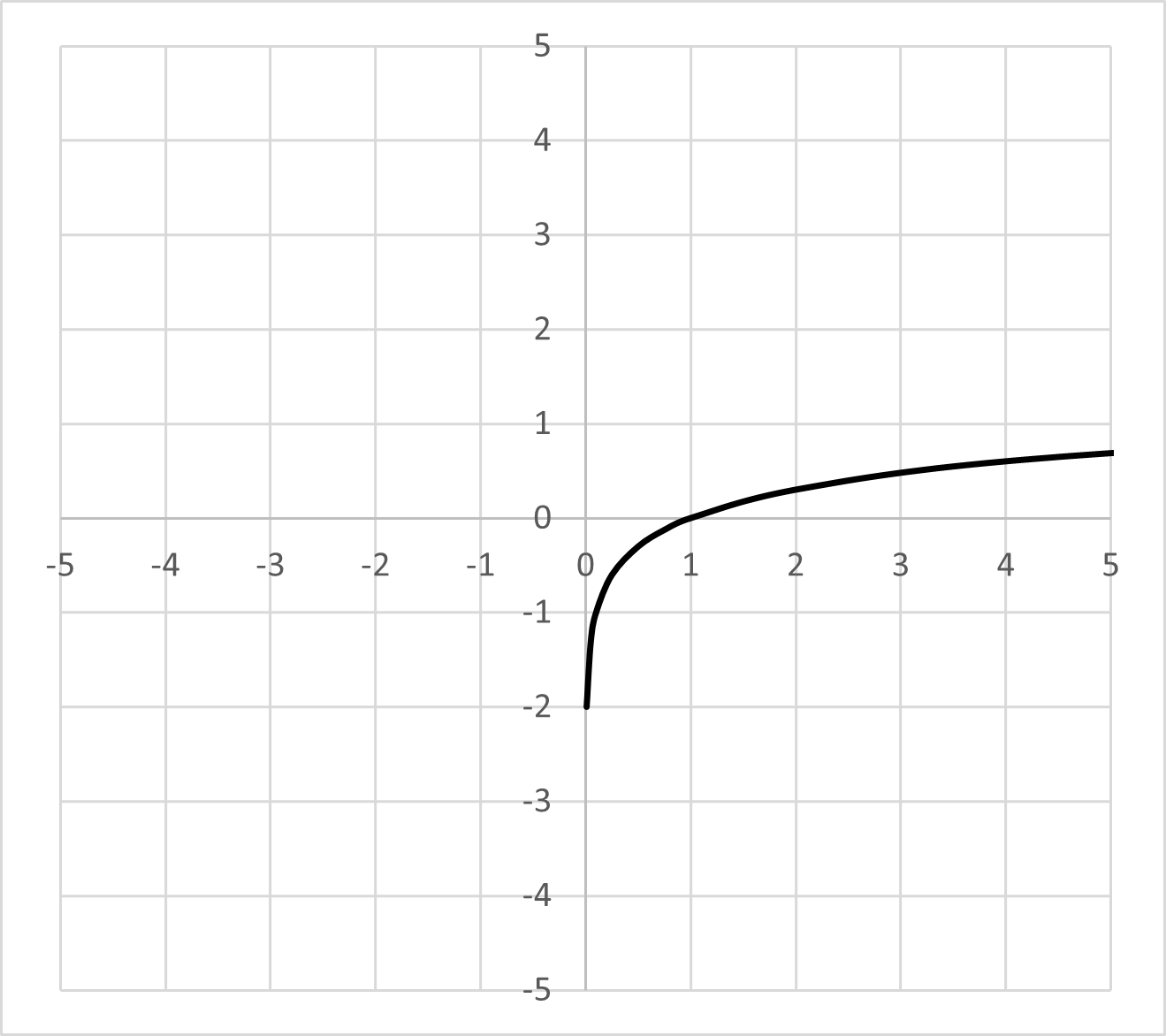
$Graph of nonlinear function logarithmic equation$

Fraction

$y = \frac{1}{x}$

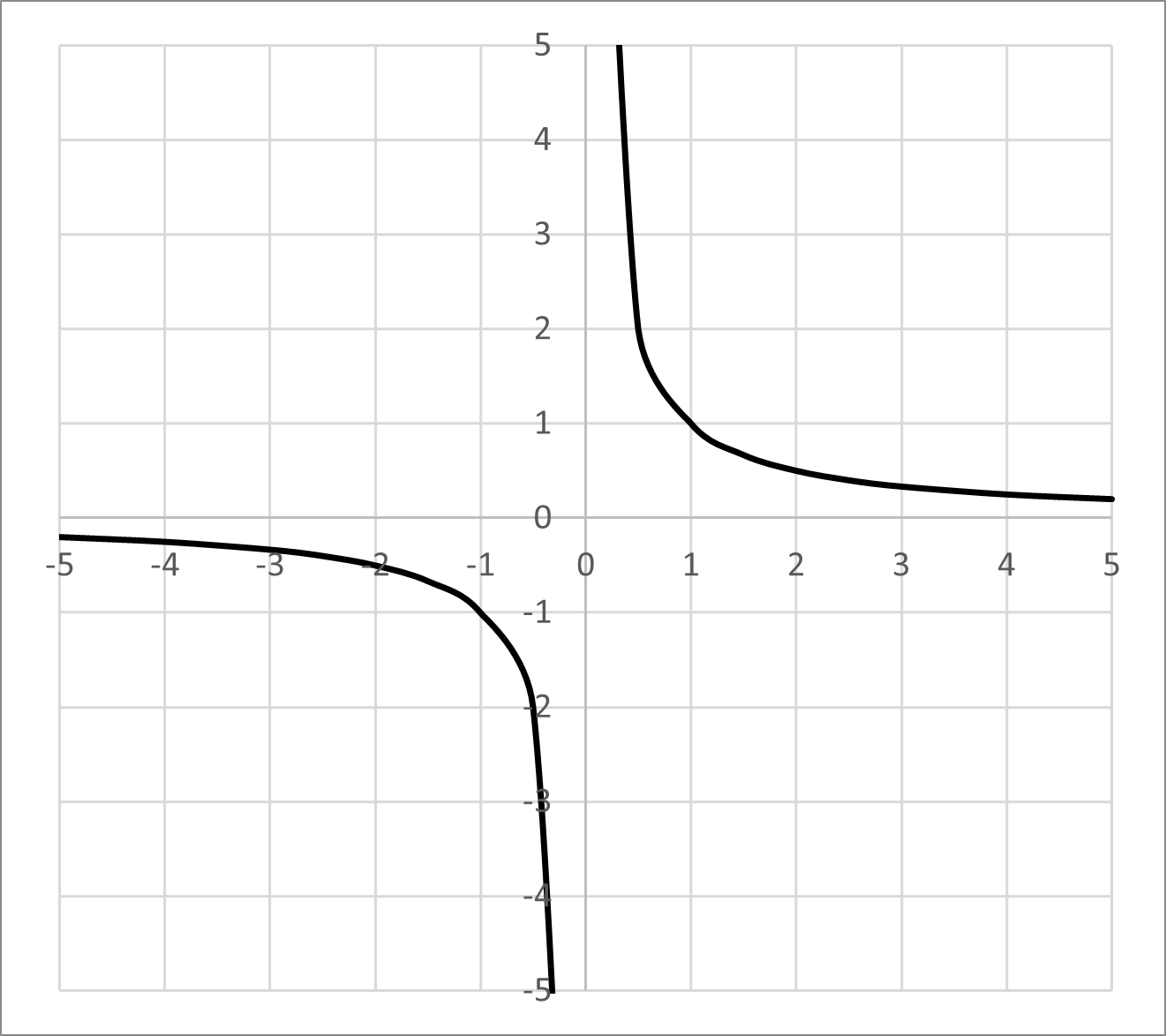
$Graph of nonlinear function fraction equation$

Square root

$y = x$

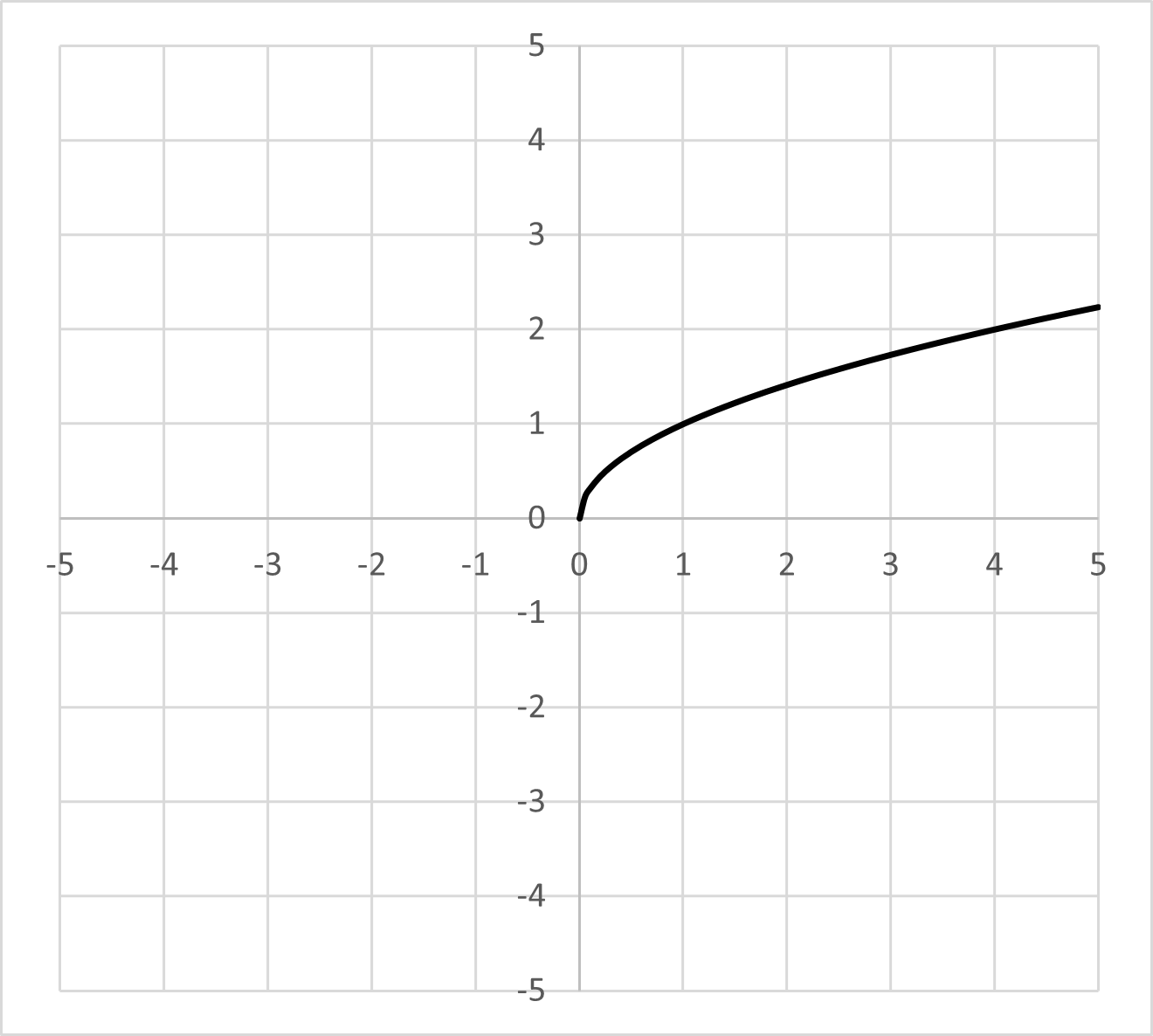
$Graph of nonlinear function square root equation$

Transformations

Most of the time, you will not be given parent functions. Instead, you will have a function that is slightly different from the parent function. We call them transformations of their parent functions, and we have some simple strategies to predict what they will look like. The most basic movements, up, down, left, and right, are called translations.

The most effective way to confirm your understanding of a transformation is to use the graphing function of a graphing calculator if you have one. However, below we will explore how to predict these graphs even without a calculator. This will help you to solve simple forms of this type of problem more quickly.

Translations—vertical

Vertical movements compared to the parent graph depend on addition or subtraction outside the function. The idea of being “outside of the function” will make more sense later on. For now, let’s see an image of a vertical translation:

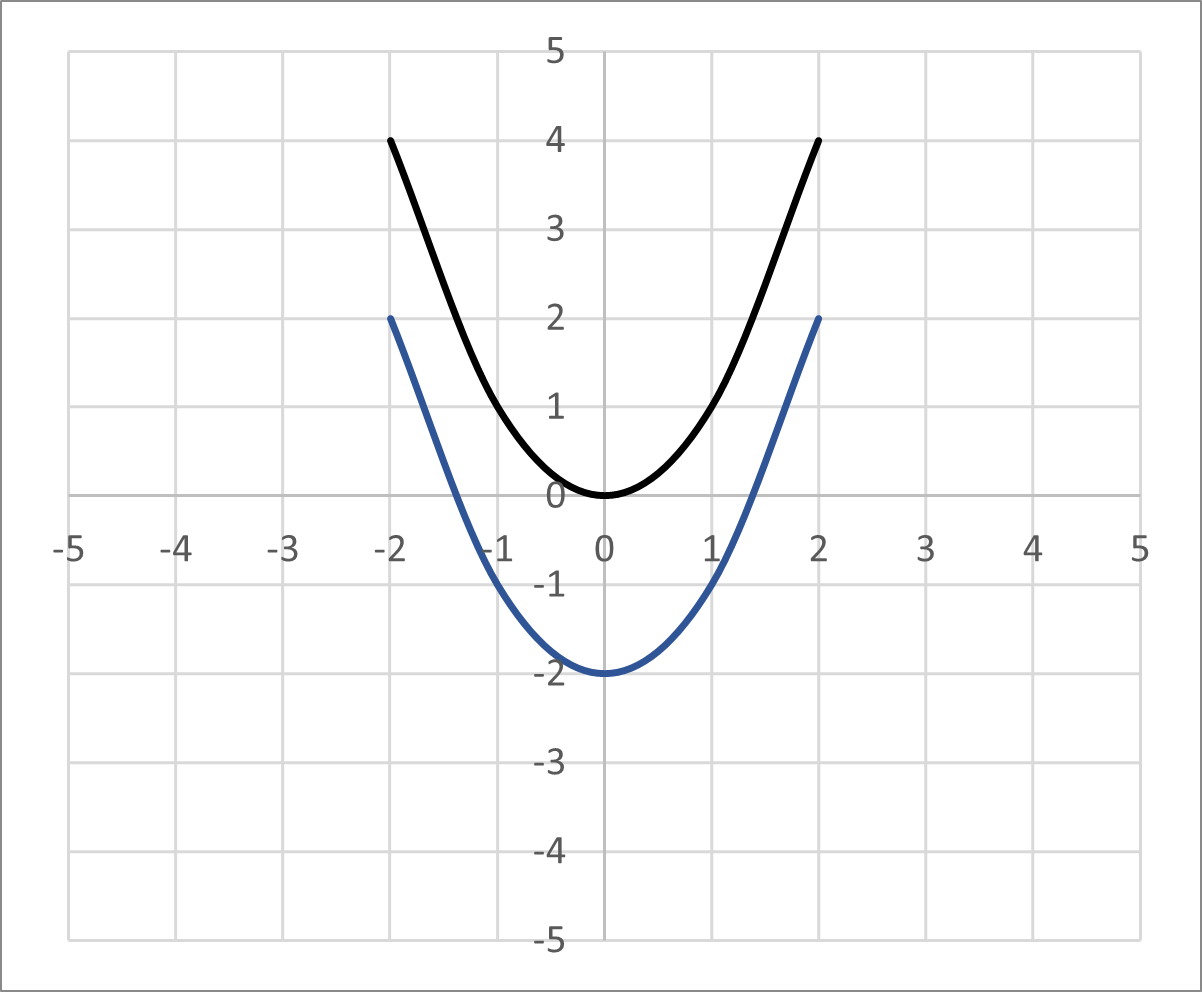
$Graph of vertical translation applied to an equation$

Since the black curve is the parent quadratic function, we know that the blue line must be the parent function after experiencing a vertical translation. In this case, the curve has shifted down $2$ units on the coordinate grid.

A downward translation occurs due to subtraction on the outside of a function. An upward movement is similarly due to addition on the outside of a function. So, the equation for the blue curve is $y = x^{2} - 2$ .

If the equation were instead $y = 3 + x^{2}$ , the transformation would be an upward translation of $3$ units. In other words, it would move up $3$ units compared to the parent function.

Translations—horizontal

Horizontal movements compared to the parent graph are caused by addition and subtraction inside the function. For a quadratic function, this means that the addition or subtraction is inside the term being squared. For a square root function, it would be within the radical. Let’s view an example of this:

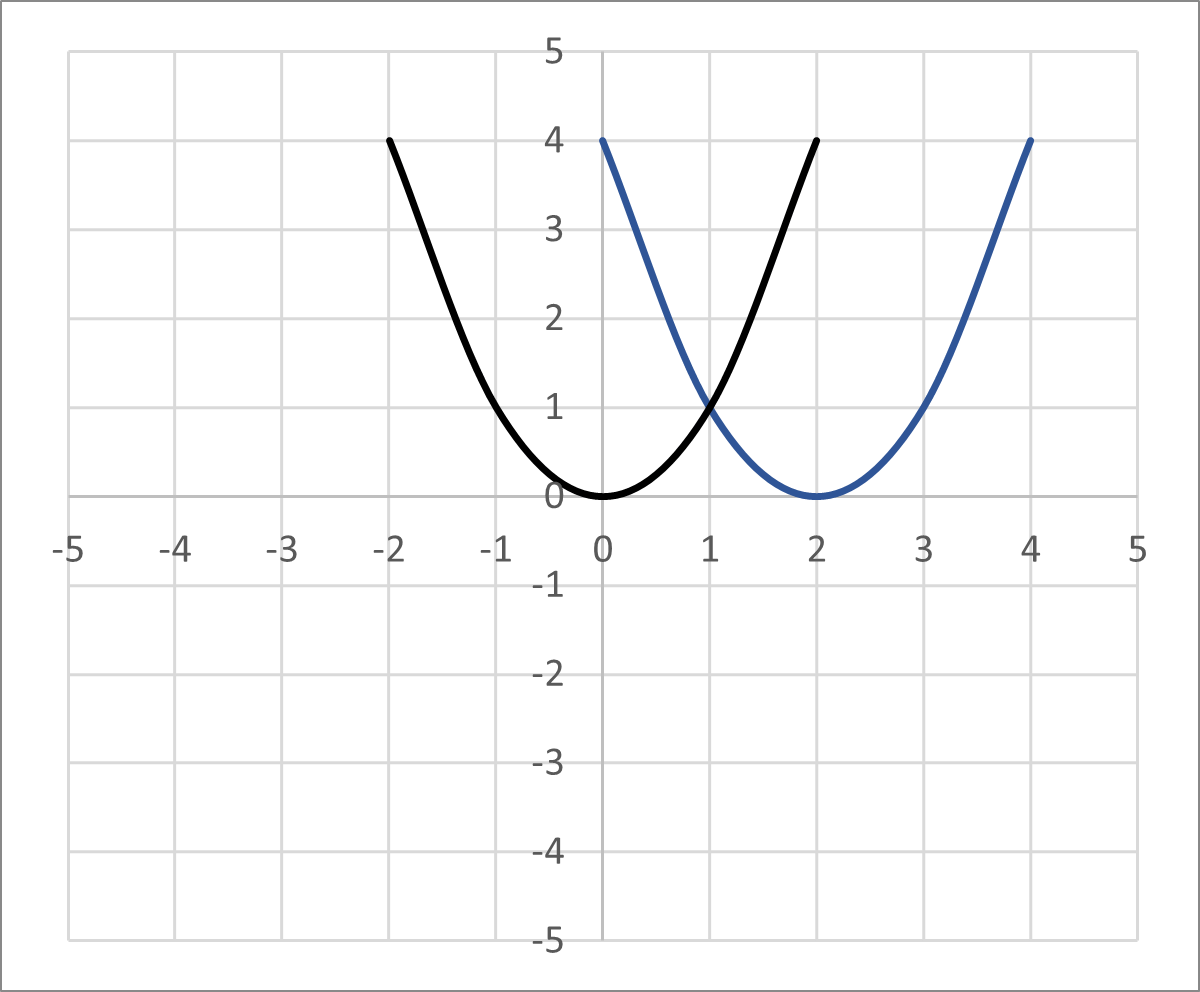
$Graph of horizontal translation applied to an equation$

The blue curve has experienced a horizontal translation of two units to the right. Its corresponding equation is $y = (x - 2)^{2}$ . This is what is meant by “inside the function.” You can see that the subtraction is within the term being squared.

A transition to the right occurs due to subtraction inside the function and a transition to the left occurs due to addition inside the function. The idea of addition pushing a graph to the left can seem counterintuitive for some people, so make sure you memorize it!

Reflection

A reflection occurs when a graph is flipped over an axis. The most common example of this is when a function is negative, causing the graph to be reflected over the $x$ -axis, like below:

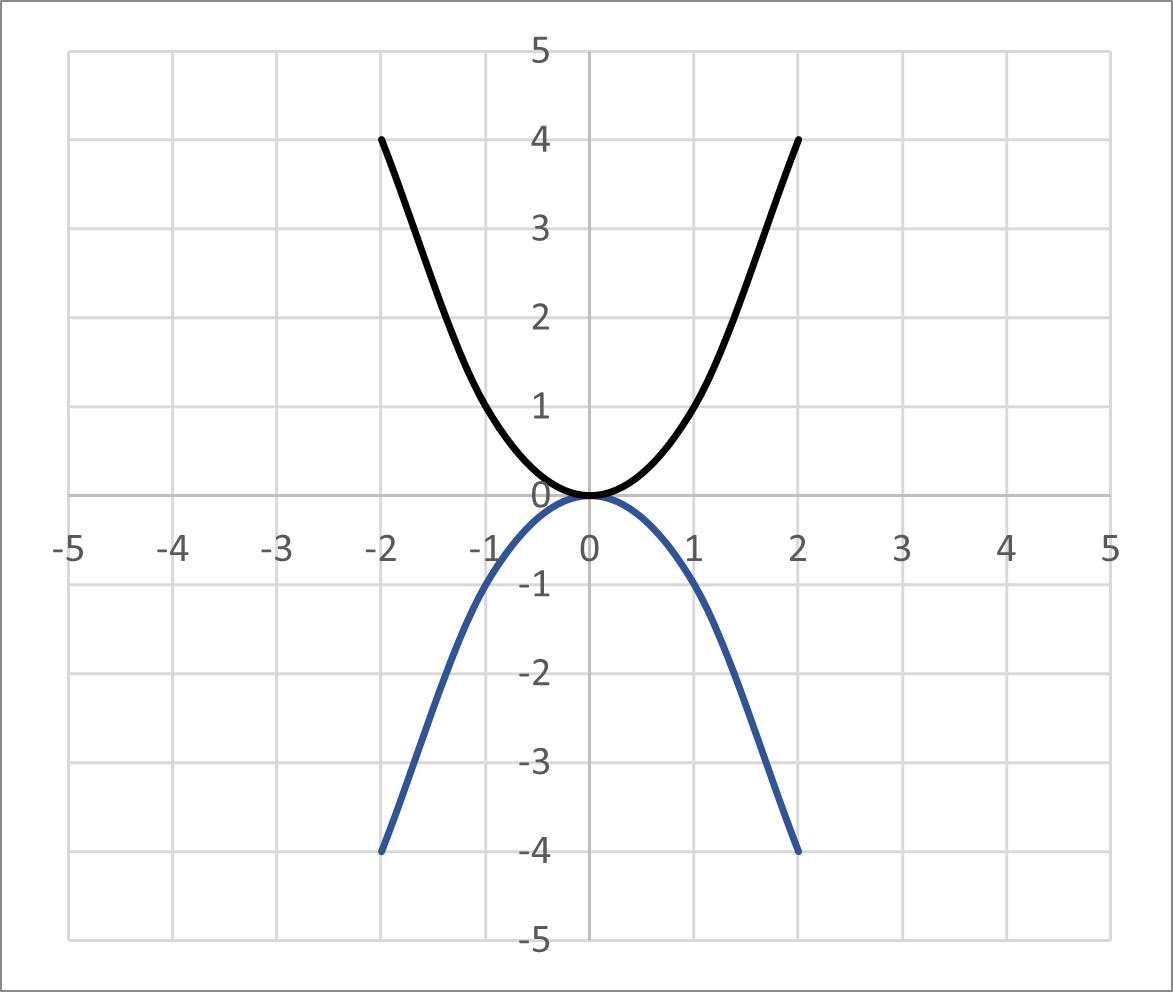
$Graph of vertical reflection across the x-axis applied to an equation$

The equation for this graph is simply the negative form of the parent quadratic function: $y = - x^{2}$ .

Asymptotes

Asymptotes are imaginary lines that mark the limitations of a graph. We can use vertical and horizontal asymptotes to describe some of the parent functions listed above and draw graphs more accurately. We can find these asymptotes by looking at the graph and, at times, by looking at the equation for a graph.

Vertical asymptotes

A vertical asymptote is an imaginary vertical line that a graph is unable to pass through. It helps us find out the behavior of a function as the input gets very large or very small. For instance, you’ll notice that the logarithmic graph takes a sharp decline the closer the $x$ value gets to $0$ , though it never actually crosses the $y$ axis. This is because there is a vertical asymptote at $x = 0$ .

To make sense of this, we need to think in terms of the function itself. Why would there be a vertical asymptote at $x = 0$ ? It occurs because the logarithm of $0$ is undefined. This means that sometimes an asymptote appears because a function would become undefined if it crossed the value where it is found.

We can find a vertical asymptote from a function by figuring out where a function is undefined. For a logarithm, it is where $x$ is equal to $0$ . Another common function to have vertical asymptotes is the fraction function. Let’s do an example below to find the vertical asymptotes of a fraction function:

What are the vertical asymptotes of the function below?

$y = \frac{( x + 3 )}{( x ^{2} - 3 x + 2 )}$

We first need to remember that a fraction is undefined when the denominator is equal to $0$ . So, our goal is to find the $x$ values that make the denominator of this fraction equal to $0$ . We can do this by factoring the quadratic equation and finding its solutions:

$y = \frac{( x + 3 )}{( x - 1 ) ( x - 2 )}$

$x = 1, x = 2$

The vertical asymptotes of this function are at $x = 1$ and $x = 2$ . We can verify this by plugging either of the values into the function for $x$ and seeing that the denominator comes out to $0$ , resulting in an undefined value.

Horizontal asymptotes

Horizontal asymptotes are the same concept as vertical asymptotes, except that they describe what happens to a function when the input gets very large or very small. An important distinction of horizontal asymptotes is that we find them primarily in fraction functions.

They are also determined in a different manner from vertical asymptotes. The way we find the value of a vertical asymptote is by comparing the highest exponential power of the numerator and denominator of a fraction function.

If the highest exponential power of the denominator is greater than that of the numerator, then the horizontal asymptote is equal to $0$ . If the highest exponential power of the numerator and denominator are equal, then the horizontal asymptote is equal to the coefficient attached to those highest power variables. If the highest power of the numerator is greater than that of the denominator, then there is no horizontal asymptote.

The table below will illustrate these conditions and a relevant example of each:

Condition (Exponential Powers)	Horizontal Asymptote (HA) Value	Example
Denominator > Numerator	HA at $y = 0$	$\frac{x}{( 1 + x ^{2} )}$ Highest powers: $\frac{x}{x ^{2}}$ HA at $y = 0$
Denominator = Numerator	HA at $y =$ variable coefficient	$\frac{( x ^{3} - x )}{( 3 x ^{3} + 2 x ^{2} + 4 )}$ Highest powers: $\frac{x ^{3}}{3 x ^{3}}$ HA at $y = \frac{1}{3}$
Denominator < Numerator	No HA	$\frac{( 2 x ^{2} + 3 x - 12 )}{( 2 x + 1 )}$ Highest powers: $\frac{2 x ^{2}}{2 x}$ No HA

Key points

Common nonlinear parent functions. Quadratic, absolute value, cubic, exponential, logarithmic, fraction, and square root functions.

Transformations. These are modifications of the parent function of a graph.

Vertical translations. These are caused by addition and subtraction outside the function. Addition moves the graph upward and subtraction moves it downward.

Horizontal translations. These are caused by addition and subtraction inside the function. Addition moves the graph to the left and subtraction moves it to the right.

Vertical asymptotes. Imaginary vertical lines that mark boundaries that a graph will not cross. These may be found by identifying the $x$ values that make a function undefined.

Horizontal asymptotes. Imaginary horizontal lines that mark boundaries that a graph will not cross. Find horizontal asymptotes by the rules for fraction exponential powers in the table above.