For the ACT test, there is a list of units that you should be familiar with:
| Category | Unit |
|---|---|
| Mass | Kilograms (kg) Grams (g) Milligrams (mg) |
| Length | Kilometers (km) Meters (m) Centimeters (cm) Millimeters (mm) Yards (yds) Feet (ft) Inches (in) |
| Volume | Liters (L) Milliliters (ml) |
In this chapter we will discuss how to go from one unit to a different unit within the same box (i.e., grams to kilograms, which are both in the “Mass” box).
Most students have more trouble converting between metric units, so that will be the main focus of this chapter. Standard units (i.e., yards, feet, and inches) should be more familiar to you, but we will take a quick look at them before moving onto the metric portion.
From smallest to largest, there are 12 inches in a foot, and 3 feet in a yard. In the following number line you can see the units from smallest to largest, and how many it takes to get to the next largest unit (represented by the smaller arrows above the number line).

How many inches are in a yard?
There are 36 inches in a yard:
This is the extent you should be comfortable with converting standard units of measurement. So remember: inches, feet, yards. There are 12 inches in a foot, 3 feet in a yard!
A good way to imagine how to convert from one unit to another is to view all of the units from one box on a number line. Below are number lines that we will reference as we discuss conversions through each box.
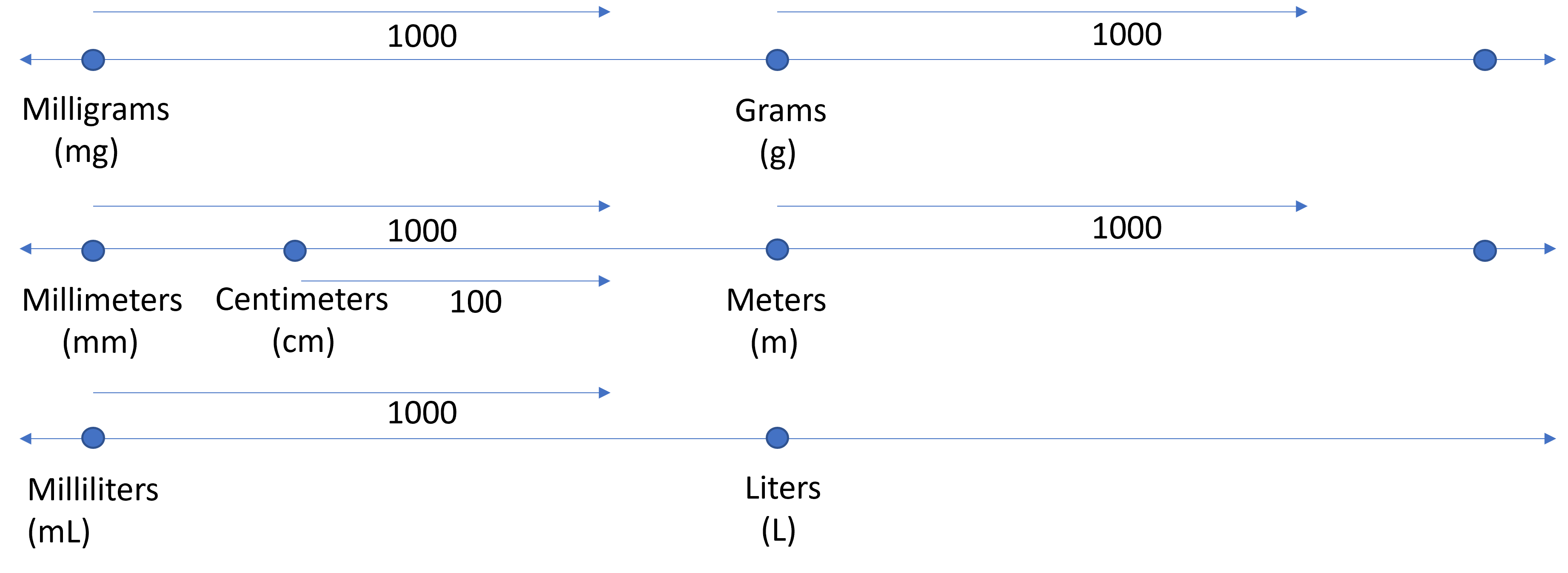
You will notice that for almost every single conversion there is a “1,000” going from one unit to the next. Just like the previous number line, this is to show that it takes 1,000 of a smaller unit to get to 1 larger unit. For instance, it takes 1,000 millimeters to create 1 meter. The only metric unit for which this is not the case is centimeters, which lies between millimeters and meters. It takes 100 cm to create one meter.
This can be easy to remember if you remember the prefixes milli- and centi- look very similar to the roots of “thousand” and “hundred”. This occurs in English words like millennium (a thousand years, or century (one hundred years), as well as Latin (milia, centum), Spanish (mil, cien), and French (mil, cent).
Once you are comfortable with how many of one unit it takes to get to the next, you can start to convert by using the number lines. Let’s say we have 30,000 milliliters and we want to show that in liters. We say, “Well, it takes 1,000 milliliters to get to 1 liter. So how many thousands do we have in thirty-thousand?”
That means that our 30,000 mL is equivalent to 30 L.
Try converting 250,000 milligrams to kilograms. How many kg do we have when we have 250,000 mg?
There are 1,000 mg in 1 g. Since , we have 250 g.
Now we need to go from g to kg, what the question wants us to finish with.
There are 1,000 g in 1 kg. , so we have 0.25 kg total in 250,000 mg.
This section is a different method for doing unit conversions. So, you can feel free to pick whichever method is easier and makes more sense for you.
The idea of T-chart unit conversions is that we will set up a T-chart and lay out all of our units so that we make sure we don’t miss any math. We will multiply all numbers on the top, multiply all numbers on the bottom, then divide the top total by the bottom total. Then, we do the math all at once as it is written down on our paper, and we will get our answer. Let’s take a look:
| How many of our first unit do we have? | 1 of our 2nd unit | 1 of our 3rd unit |
|---|---|---|
| How many of 1st unit does it take to get to 2nd unit? | How many of 2nd unit does it take to get to 3rd unit? |
Starting with our first unit on the top left, we make sure that when we go to the second column our 1st unit boxes are diagonal from each other. This way the units “cancel out” because we divide the unit by itself. If we are solving for the second unit, then we can stop there (i.e., going from mg to g, which is just one level on the number line above mg). If we are going to a 3rd unit, or another level above (i.e., going from mg to g, then going from g to kg), then we will do the same thing, making sure the 2nd unit boxes are diagonal from each other. Then we will end up with the 3rd unit as our answer.
Take a look at an example converting from 3,000,000 mm to km, then we will examine it a little further so that it makes more sense:
| 3,000,000 mm | 1 m | 1 km |
|---|---|---|
| 1,000 mm | 1,000 m |
Let’s go through some steps:
Write what we have in the top left
Write into the box diagonal to the bottom-left how many of the 1st unit it takes to get to 1 of the next unit
Write 1 of the 2nd unit in the top of the 2nd column
This second column, for instance, shows that there are 1,000 mm in 1 m. The reason why mm is on the bottom is so that it is diagonal to the mm on the top-left. This shows that when we divide 3,000,000 mm by 1,000 mm, the mm unit will “cancel out”.
Repeat these steps with another column if you need to keep converting to a larger unit
When we have everything written out,
Multiply everything on the top together
Multiply everything on the bottom together
Then, take the total number on top and divide by the total number on bottom
The number you get will be your final answer
Important to note: the unit of your final answer will be the top-right unit in the table (it is not crossed out by having a similar unit diagonal to it)
Remember, you don’t have to use this technique. Oftentimes, this is difficult for most students to understand. However, it is a great skill, and can keep you from getting confused about whether you need to multiply or divide. So, use whichever method you are most comfortable with to convert between units!
Something you may notice about questions involving the area of a shape is that the units of area are given to you in (i.e., , , square yards).
This is because to find an area you take one side (a unit of length like inches) and multiply it by the other side (also a unit of length like inches). So, .
You need to keep this in mind when you convert units of area. All of your values in your conversions must be squared when using square units. Follow this example:
| () | () |
All of our normal conversion units remain the same, but we have to square them all as you can see. This is the only difference you need to make when converting units of area, but you will need to keep an eye out for this difference.
Sign up for free to take 3 quiz questions on this topic